Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
5. Graphical Applications of Derivatives
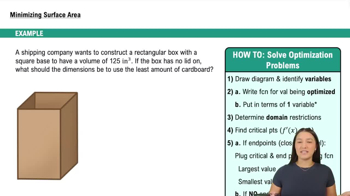
Applied Optimization
Problem 4.8.37b
Textbook Question
{Use of Tech} A damped oscillator The displacement of an object as it bounces vertically up and down on a spring is given by y(t) = 2.5e⁻ᵗ cos 2t, where the initial displacement is y(0) = 2.5 and y = 0 corresponds to the rest position (see figure). <IMAGE>
b. Find the time and the displacement when the object reaches its lowest point.
 Verified step by step guidance
Verified step by step guidance1
To find the time when the object reaches its lowest point, we need to find the critical points of the function y(t) = 2.5e^(-t) cos(2t). This involves taking the derivative of y(t) with respect to t.
Apply the product rule to differentiate y(t) = 2.5e^(-t) cos(2t). The product rule states that if you have a function h(t) = u(t)v(t), then h'(t) = u'(t)v(t) + u(t)v'(t). Here, u(t) = 2.5e^(-t) and v(t) = cos(2t).
Differentiate u(t) = 2.5e^(-t) to get u'(t) = -2.5e^(-t). Differentiate v(t) = cos(2t) to get v'(t) = -2sin(2t) using the chain rule.
Substitute the derivatives into the product rule: y'(t) = (-2.5e^(-t))cos(2t) + (2.5e^(-t))(-2sin(2t)). Simplify this expression to find y'(t).
Set y'(t) = 0 to find the critical points. Solve the resulting equation for t to find the times when the object reaches its lowest point. Evaluate y(t) at these times to find the corresponding displacements.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Damped Oscillator
A damped oscillator is a system in which the amplitude of oscillation decreases over time due to energy loss, often from friction or resistance. The displacement function typically includes an exponential decay factor, which represents this loss of energy. In the given equation, the term '2.5e⁻ᵗ' indicates that the oscillation's amplitude diminishes as time progresses.
Recommended video:

Cases Where Limits Do Not Exist
Cosine Function in Oscillations
The cosine function is fundamental in describing periodic motion, such as oscillations. In the equation y(t) = 2.5e⁻ᵗ cos 2t, the 'cos 2t' part represents the oscillatory behavior of the system, where '2t' indicates the frequency of oscillation. The cosine function oscillates between -1 and 1, determining the position of the object at any given time.
Recommended video:

Graph of Sine and Cosine Function
Finding Extrema
To find the lowest point of the oscillation, we need to determine the extrema of the displacement function. This involves taking the derivative of y(t) with respect to time, setting it to zero to find critical points, and then evaluating these points to identify the minimum displacement. The lowest point corresponds to the maximum negative value of the displacement function.
Recommended video:
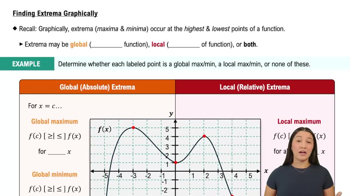
Finding Extrema Graphically

 1:13m
1:13mWatch next
Master Intro to Applied Optimization: Maximizing Area with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice