Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
5. Graphical Applications of Derivatives
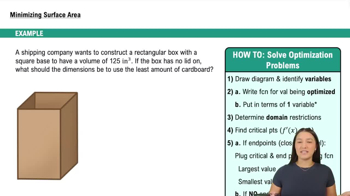
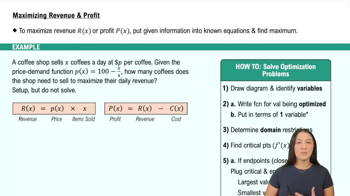
Applied Optimization
Problem 4.8.37c
Textbook Question
{Use of Tech} A damped oscillator The displacement of an object as it bounces vertically up and down on a spring is given by y(t) = 2.5e⁻ᵗ cos 2t, where the initial displacement is y(0) = 2.5 and y = 0 corresponds to the rest position (see figure). <IMAGE>
c. Find the time at which the object passes the rest position for the second time.
 Verified step by step guidance
Verified step by step guidance1
First, understand the function y(t) = 2.5e⁻ᵗ cos 2t. This represents a damped oscillator, where the term 2.5e⁻ᵗ is the damping factor and cos 2t is the oscillatory component.
To find when the object passes the rest position, set y(t) = 0 and solve for t. This means solving the equation 2.5e⁻ᵗ cos 2t = 0.
Since 2.5e⁻ᵗ is never zero for any real value of t, focus on the oscillatory part: cos 2t = 0. The cosine function is zero at odd multiples of π/2, so set 2t = (2n+1)π/2, where n is an integer.
Solve for t: t = (2n+1)π/4. This gives the times at which the object passes the rest position.
To find the second time the object passes the rest position, use n = 1 (since n = 0 corresponds to the first time). Substitute n = 1 into the equation t = (2n+1)π/4 to find the second time.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
5mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Damped Oscillator
A damped oscillator is a system in which the amplitude of oscillation decreases over time due to energy loss, often modeled by an exponential decay function. In the given equation, the term '2.5e⁻ᵗ' represents this damping effect, indicating that the displacement diminishes as time progresses.
Recommended video:

Cases Where Limits Do Not Exist
Cosine Function in Oscillations
The cosine function is fundamental in describing periodic motion, such as oscillations. In the equation y(t) = 2.5e⁻ᵗ cos 2t, the 'cos 2t' component indicates the oscillatory behavior of the system, with '2' representing the angular frequency, which affects the speed of oscillation.
Recommended video:

Graph of Sine and Cosine Function
Finding Roots of the Function
To determine when the object passes the rest position (y = 0), we need to find the roots of the function y(t). This involves solving the equation 2.5e⁻ᵗ cos 2t = 0, which occurs when cos 2t = 0, leading to specific values of t that correspond to the times the object crosses the rest position.
Recommended video:

Finding Limits by Direct Substitution

 1:13m
1:13mWatch next
Master Intro to Applied Optimization: Maximizing Area with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice