Use a calculator to approximate each value in decimal degrees.
θ = cos⁻¹ 0.80396577
 Lial 12th Edition
Lial 12th Edition Ch. 6 - Inverse Circular Functions and Trigonometric Equations
Ch. 6 - Inverse Circular Functions and Trigonometric Equations Problem 6.21
Problem 6.21 Verified step by step guidance
Verified step by step guidance
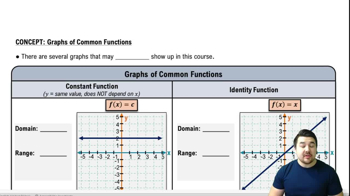


Use a calculator to approximate each value in decimal degrees.
θ = cos⁻¹ 0.80396577
Solve each equation for x, where x is restricted to the given interval.
y = cos (x + 3) , for x in [―3, π―3]
Find the exact value of each real number y if it exists. Do not use a calculator.
y = arcsin (―√3/2)
Solve each equation for x, where x is restricted to the given interval.
y = √2 + 3 sec 2x, for x in [0, π/4) ⋃ (π/4, π/2]
Find the exact value of each real number y if it exists. Do not use a calculator.
y = arccos (―√3/2)
Evaluate each expression without using a calculator.
cos (arccos (-1))