Which one of the following equations has solution 0?
a. arctan 1 = x
b. arccos 0 = x
c. arcsin 0 = x
 Lial 12th Edition
Lial 12th Edition Ch. 6 - Inverse Circular Functions and Trigonometric Equations
Ch. 6 - Inverse Circular Functions and Trigonometric Equations Problem 6.11
Problem 6.11 Verified step by step guidance
Verified step by step guidance

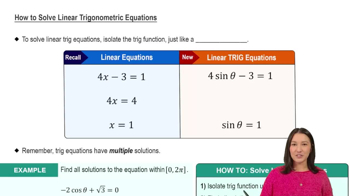

Which one of the following equations has solution 0?
a. arctan 1 = x
b. arccos 0 = x
c. arcsin 0 = x
Write each trigonometric expression as an algebraic expression in u, for u > 0.
tan (sin⁻¹ u/(√u² + 2))
Write each trigonometric expression as an algebraic expression in u, for u > 0.
sec (arccot (√4―u² )/ u)
Find the exact value of each real number y. Do not use a calculator.
y = sec⁻¹ (―2)
Solve each equation for x, where x is restricted to the given interval.
y = ― 2 cos 5x , for x in [0, π/5]
Find the exact value of each real number y if it exists. Do not use a calculator.
y = sin⁻¹ 0