Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
3. Unit Circle
Reciprocal Trigonometric Functions on the Unit Circle
Problem 26a
Textbook Question
Textbook QuestionIn Exercises 25–32, the unit circle has been divided into eight equal arcs, corresponding to t-values of
0, 𝜋, 𝜋, 3𝜋, 𝜋, 5𝜋, 3𝜋, 7𝜋, and 2𝜋.
4 2 4 4 2 4
a. Use the (x,y) coordinates in the figure to find the value of the trigonometric function.
cos 3𝜋/4
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Unit Circle
The unit circle is a circle with a radius of one centered at the origin of a coordinate plane. It is fundamental in trigonometry as it provides a geometric representation of the sine and cosine functions. The coordinates of any point on the unit circle correspond to the cosine and sine of the angle formed with the positive x-axis, allowing for easy calculation of trigonometric values.
Recommended video:

Introduction to the Unit Circle
Trigonometric Functions
Trigonometric functions, such as sine, cosine, and tangent, relate the angles of a triangle to the lengths of its sides. In the context of the unit circle, the cosine of an angle is the x-coordinate of the corresponding point on the circle, while the sine is the y-coordinate. Understanding these functions is crucial for evaluating trigonometric expressions and solving problems involving angles.
Recommended video:

Introduction to Trigonometric Functions
Reference Angles
Reference angles are the acute angles formed by the terminal side of an angle and the x-axis. They help simplify the evaluation of trigonometric functions for angles greater than 90 degrees or less than 0 degrees. For example, to find cos(3π/4), one can use the reference angle π/4, which allows for easier calculation by recognizing the cosine's sign in the second quadrant.
Recommended video:

Reference Angles on the Unit Circle

 3:23m
3:23mWatch next
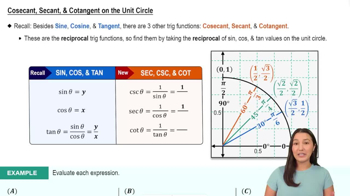
Master Secant, Cosecant, & Cotangent on the Unit Circle with a bite sized video explanation from Callie Rethman
Start learningRelated Videos
Related Practice