Here are the essential concepts you must grasp in order to answer the question correctly.
Electric Field
The electric field is a vector field that represents the force exerted by an electric charge on other charges in its vicinity. It is defined as the force per unit charge and is measured in newtons per coulomb (N/C). For a uniformly charged sphere, the electric field outside the sphere behaves as if all the charge were concentrated at the center, allowing for straightforward calculations using Gauss's law.
Recommended video:
Volume Charge Density
Volume charge density, denoted by ρ, is a measure of the amount of electric charge per unit volume within a given region of space. It is expressed in coulombs per cubic meter (C/m³). For a uniformly charged insulating sphere, the volume charge density can be calculated by dividing the total charge by the volume of the sphere, which is given by the formula V = (4/3)πR³.
Recommended video:
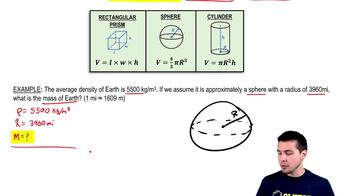
Problems with Mass, Volume, & Density
Gauss's Law
Gauss's Law relates the electric flux through a closed surface to the charge enclosed by that surface. Mathematically, it states that the total electric flux is equal to the enclosed charge divided by the permittivity of free space. This law is particularly useful for calculating electric fields in symmetric charge distributions, such as spheres, as it simplifies the process of finding the electric field at a distance from the charge.
Recommended video: