Calculate the moment of inertia of each of the following uniform objects about the axes indicated. Consult Table 9.2 as needed. (a) A thin 2.50-kg rod of length 75.0 cm, about an axis perpendicular to it and passing through (i) one end and (ii) its center, and (iii) about an axis parallel to the rod and passing through it.

Verified step by step guidance

1. Identify the shape and mass distribution of the object. In this case, the object is a thin rod with a uniform mass distribution.
2. Use the formula for the moment of inertia of a thin rod about an axis perpendicular to it and passing through one end: $I = \frac{1}{3} M L^2$, where $M$ is the mass of the rod and $L$ is its length.
3. Use the formula for the moment of inertia of a thin rod about an axis perpendicular to it and passing through its center: $I = \frac{1}{12} M L^2$.
4. For the moment of inertia about an axis parallel to the rod and passing through it, use the formula $I = \frac{1}{12} M L^2$ since the axis is along the length of the rod and the rod's radius is negligible.
5. Convert all lengths from centimeters to meters before substituting into the formulas to ensure consistency in units.

Key Concepts

Moment of Inertia

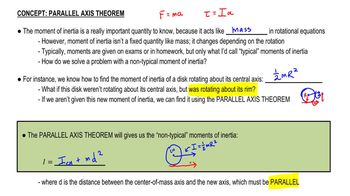
The moment of inertia is a measure of an object's resistance to rotational motion about a specific axis. It depends on the mass distribution relative to that axis, with greater distances from the axis resulting in a higher moment of inertia. The formula for calculating it varies based on the shape and mass distribution of the object, and it is crucial for understanding how objects behave when subjected to rotational forces.

Axis of Rotation

The axis of rotation is an imaginary line around which an object rotates. The choice of this axis significantly affects the moment of inertia, as it determines how the mass of the object is distributed in relation to that line. In the given question, different axes (one end, center, and parallel to the rod) will yield different moments of inertia for the same object, illustrating the importance of the axis in rotational dynamics.

Uniform Objects

Uniform objects have a consistent mass distribution throughout their volume, which simplifies the calculation of their moment of inertia. For example, a uniform rod has the same density along its length, allowing for straightforward application of standard formulas found in reference tables. Understanding the properties of uniform objects is essential for accurately determining their rotational characteristics.