Here are the essential concepts you must grasp in order to answer the question correctly.
Moment of Inertia
The moment of inertia is a measure of an object's resistance to changes in its rotational motion about a specific axis. It depends on the mass distribution relative to that axis. For composite objects, the total moment of inertia is the sum of the moments of inertia of individual components, calculated using their respective mass and distance from the axis of rotation.
Recommended video:
Intro to Moment of Inertia
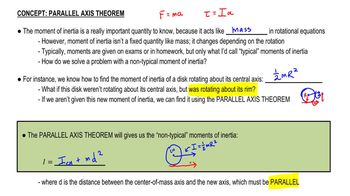
Parallel Axis Theorem
The parallel axis theorem allows for the calculation of the moment of inertia of an object about any axis, given its moment of inertia about a parallel axis through its center of mass. It states that the moment of inertia about the new axis is equal to the moment of inertia about the center of mass plus the product of the mass and the square of the distance between the two axes.
Recommended video:
Composite Bodies
Composite bodies consist of multiple shapes or components, each contributing to the overall moment of inertia. In the case of the wagon wheel, the rim and spokes are treated as separate components, and their individual moments of inertia are calculated and summed to find the total moment of inertia of the wheel. Understanding how to analyze each part is crucial for accurate calculations.
Recommended video:
Vector Composition & Decomposition