flat (unbanked) curve on a highway has a radius of 170.0 m. A car rounds the curve at a speed of 25.0 m/s. (a) What is the minimum coefficient of static friction that will prevent sliding?

Verified step by step guidance

1. Identify the forces acting on the car: Since the car is moving in a circular path, there is a centripetal force required to keep the car moving in that path. This force is provided by the friction between the car's tires and the road.
2. Use the formula for centripetal force, which is given by \( F_c = \frac{mv^2}{r} \), where \( m \) is the mass of the car, \( v \) is the speed of the car, and \( r \) is the radius of the curve.
3. Recognize that the frictional force must be equal to the centripetal force to prevent the car from sliding. Thus, \( f = F_c \).
4. The maximum frictional force that can act without sliding is given by \( f_{\text{max}} = \mu_s N \), where \( \mu_s \) is the coefficient of static friction and \( N \) is the normal force. In this case, the normal force is equal to the gravitational force, \( N = mg \), where \( g \) is the acceleration due to gravity.
5. Set up the equation \( \mu_s mg = \frac{mv^2}{r} \) and solve for \( \mu_s \) by dividing both sides by \( mg \), giving \( \mu_s = \frac{v^2}{rg} \). This will provide the minimum coefficient of static friction needed to prevent the car from sliding.

Key Concepts

Centripetal Force

Centripetal force is the net force required to keep an object moving in a circular path, directed towards the center of the circle. For a car rounding a curve, this force is provided by the friction between the tires and the road. The formula for centripetal force is F_c = (mv^2)/r, where m is the mass of the car, v is its speed, and r is the radius of the curve.

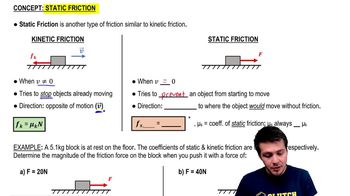
Static Friction

Static friction is the force that resists the initiation of sliding motion between two surfaces in contact. It is crucial for a car to maintain its path on a curve without sliding off. The maximum static frictional force can be calculated using the equation F_friction = μ_s * N, where μ_s is the coefficient of static friction and N is the normal force, which equals the weight of the car on a flat surface.

Coefficient of Static Friction

The coefficient of static friction (μ_s) is a dimensionless value that represents the ratio of the maximum static frictional force to the normal force acting between two surfaces. It indicates how much frictional force is available to prevent sliding. To find the minimum coefficient of static friction required to prevent a car from sliding while rounding a curve, one can rearrange the equations of motion and friction to solve for μ_s.