Here are the essential concepts you must grasp in order to answer the question correctly.
Acceleration
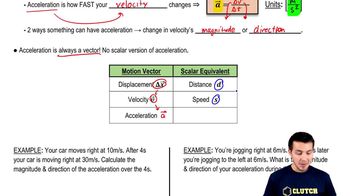
Acceleration is the rate of change of velocity of an object over time. It is a vector quantity, meaning it has both magnitude and direction. In this context, we can calculate the acceleration of the Genesis spacecraft during its crash by using the initial and final velocities, along with the time taken for the crash. The formula used is a = (v_f - v_i) / t, where v_f is the final velocity, v_i is the initial velocity, and t is the time.
Recommended video:
Kinematics
Kinematics is the branch of mechanics that deals with the motion of objects without considering the forces that cause the motion. It involves concepts such as displacement, velocity, and acceleration. In this problem, kinematic equations can be applied to relate the initial and final velocities, the distance traveled, and the acceleration to find the unknowns during the crash of the spacecraft.
Recommended video:
Gravitational Acceleration
Gravitational acceleration, denoted as 'g', is the acceleration due to Earth's gravity, approximately 9.81 m/s². In this problem, the acceleration of the spacecraft during the crash can be expressed in terms of g's by dividing the calculated acceleration by 9.81 m/s². This provides a more intuitive understanding of the acceleration experienced by the spacecraft relative to the force of gravity.
Recommended video:
Weight Force & Gravitational Acceleration