Here are the essential concepts you must grasp in order to answer the question correctly.
Position Function
The position function describes the location of an object over time. In this case, the turtle's position is given by the equation x(t) = 50.0 cm + (2.00 cm/s)t − (0.0625 cm/s²)t², which indicates that the turtle starts at 50.0 cm and moves with an initial velocity of 2.00 cm/s while experiencing a negative acceleration. Understanding this function is crucial for determining how the turtle's position changes as time progresses.
Recommended video:
Velocity
Velocity is the rate of change of position with respect to time and can be derived from the position function. It is represented as υx(t) = dx/dt, which can be calculated by differentiating the position function. For the turtle, this will yield a linear equation that shows how its speed changes over time, reflecting both the initial velocity and the effect of acceleration.
Recommended video:
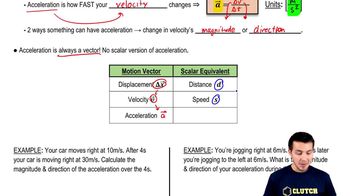
Acceleration
Acceleration is the rate of change of velocity with respect to time. In this scenario, the turtle's acceleration is constant and can be found by differentiating the velocity function. The negative term in the position equation indicates that the turtle is decelerating, which will be represented as a constant value in the acceleration graph, showing how the turtle's speed decreases over the specified time interval.
Recommended video:
 Verified step by step guidance
Verified step by step guidance