Here are the essential concepts you must grasp in order to answer the question correctly.
Density
Density is defined as mass per unit volume and is a crucial property of materials. It is calculated using the formula density = mass/volume. In this context, the density of Styrofoam (150 kg/m³) indicates how much mass is contained in a given volume, which helps determine how much weight the sphere can support before it sinks.
Recommended video:
Buoyancy
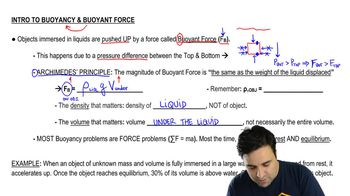
Buoyancy is the upward force exerted by a fluid that opposes the weight of an object submerged in it. According to Archimedes' principle, the buoyant force on an object is equal to the weight of the fluid displaced by the object. This principle is essential for understanding how the Styrofoam sphere can float and how much mass it can support without sinking.
Recommended video:
Intro to Buoyancy & Buoyant Force
Volume of a Sphere
The volume of a sphere is calculated using the formula V = (4/3)πr³, where r is the radius. For a 50-cm-diameter sphere, the radius is 25 cm (0.25 m). Knowing the volume allows us to determine the weight of the water displaced by the sphere, which is necessary to calculate the maximum mass that can hang from it without causing it to sink.
Recommended video:
 Verified step by step guidance
Verified step by step guidance