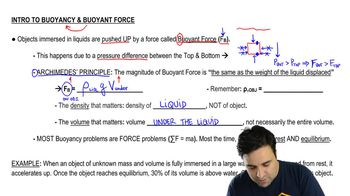
Textbook Question
A friend asks you how much pressure is in your car tires. You know that the tire manufacturer recommends 30 psi, but it's been a while since you've checked. You can't find a tire gauge in the car, but you do find the owner's manual and a ruler. Fortunately, you've just finished taking physics, so you tell your friend, 'I don't know, but I can figure it out.' From the owner's manual you find that the car's mass is 1500 kg. It seems reasonable to assume that each tire supports one-fourth of the weight. With the ruler you find that the tires are 15 cm wide and the flattened segment of the tire in contact with the road is 13 cm long. What answer—in psi—will you give your friend?
582
views