Here are the essential concepts you must grasp in order to answer the question correctly.
Work-Energy Principle
The Work-Energy Principle states that the work done on an object is equal to the change in its kinetic energy. In this scenario, the work done by the tension in the rope will increase Paul's kinetic energy as he is pulled across the mat. This principle allows us to relate the force applied, the distance moved, and the resulting speed of the object.
Recommended video:
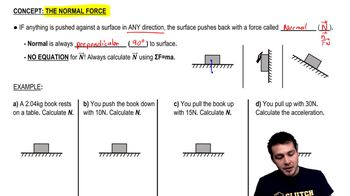
Friction and Normal Force
Friction is a force that opposes the motion of an object and is dependent on the normal force and the coefficient of friction. In this case, the normal force acting on Paul is affected by both his weight and the vertical component of the tension in the rope. Understanding how to calculate the net force considering friction is crucial for determining the net work done on Paul.
Recommended video:
Components of Forces
When dealing with forces at an angle, it is essential to resolve them into their horizontal and vertical components. The tension in the rope can be broken down into a horizontal component that contributes to pulling Paul forward and a vertical component that affects the normal force. This decomposition is vital for accurately calculating the net force and the work done on Paul.
Recommended video:
Vector Addition By Components