Here are the essential concepts you must grasp in order to answer the question correctly.
Circular Motion
Circular motion refers to the movement of an object along the circumference of a circle or a circular path. In the context of walking, the body pivots around a point (the foot) while the center of mass moves in a circular arc. This motion is characterized by centripetal acceleration, which is directed towards the center of the circular path, and is essential for understanding how forces act on the body during walking.
Recommended video:
Center of Mass
The center of mass is the point in a body or system where the mass is evenly distributed in all directions. For a human, this point is typically located near the hips. Understanding the center of mass is crucial for analyzing balance and stability during motion, as it influences how forces, such as gravity and normal force, affect the body's movement and ability to walk or run.
Recommended video:
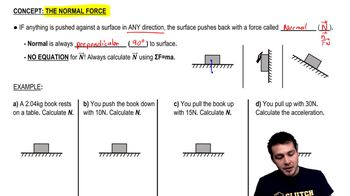
Normal Force
The normal force is the perpendicular force exerted by a surface to support the weight of an object resting on it. In walking, as a person shifts their weight and pivots over their forward foot, the normal force changes, affecting the stability and motion of the body. A decrease in normal force can lead to a loss of contact with the ground, which is why walking speed has an upper limit before transitioning to running.
Recommended video: