So-called volcanic 'ash' is actually finely pulverized rock blown high into the atmosphere. A typical ash particle is a 50-micrometer-diameter piece of silica with a density of 2400 kg/m^3. (a) How long would it take this ash particle to fall from a height of 5.0 km in vacuum?

Key Concepts

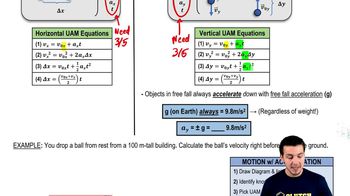
Free Fall

Free fall refers to the motion of an object under the influence of gravity alone, without any air resistance. In a vacuum, all objects fall at the same rate regardless of their mass, which is approximately 9.81 m/s² on Earth. This principle allows us to calculate the time it takes for an object to fall from a certain height using the equation of motion.

Kinematic Equations

Kinematic equations describe the motion of objects under constant acceleration. For free fall, the relevant equation is h = 0.5 * g * t², where h is the height, g is the acceleration due to gravity, and t is the time of fall. This equation can be rearranged to solve for time when the height and acceleration are known.

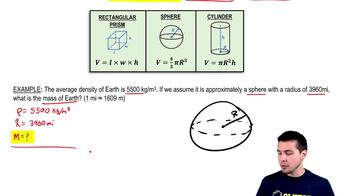
Density and Volume

Density is defined as mass per unit volume and is crucial for understanding the properties of materials. In this context, the density of the ash particle (2400 kg/m³) helps characterize its mass, but since the question specifies a vacuum, the density does not affect the fall time. Instead, it highlights the particle's physical characteristics, which are relevant in other contexts, such as sedimentation.