Here are the essential concepts you must grasp in order to answer the question correctly.
Rolling Friction
Rolling friction is the resistance that occurs when an object rolls over a surface. It is generally less than sliding friction and is influenced by factors such as the surface texture and the material of the rolling object. In this scenario, the coefficient of rolling friction (0.10) indicates how much force opposes the motion of the toy train as it rolls around the track.
Recommended video:
Conservation of Energy in Rolling Motion
Angular Speed
Angular speed refers to the rate at which an object rotates around an axis, measured in revolutions per minute (rpm) or radians per second. In this question, the toy train is released with an angular speed of 30 rpm, which needs to be converted into a more usable unit for calculations, such as radians per second, to analyze its motion and eventual stopping time.
Recommended video:
Speed Distribution & Special Speeds of Ideal Gases
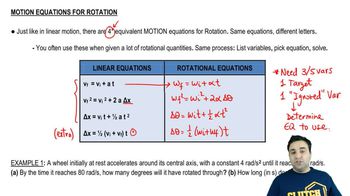
Kinematics of Rotational Motion
Kinematics of rotational motion involves the equations and principles that describe the motion of rotating objects. It includes concepts such as angular displacement, angular velocity, and angular acceleration. To determine how long it takes for the toy train to stop, one must apply these principles, particularly focusing on how the rolling friction affects the angular deceleration of the train.
Recommended video:
Equations of Rotational Motion