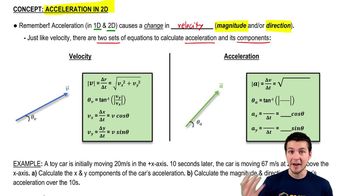
Here are the essential concepts you must grasp in order to answer the question correctly.
Centripetal Acceleration
Centripetal acceleration is the acceleration directed towards the center of a circular path that keeps an object moving in that path. It is calculated using the formula a_c = v^2 / r, where v is the tangential speed and r is the radius of the circular path. In this scenario, as the car speeds up, its tangential speed increases, affecting the centripetal acceleration.
Recommended video:
Intro to Centripetal Forces
Tangential Acceleration
Tangential acceleration refers to the rate of change of the speed of an object moving along a circular path. It is constant in this case, given as 1.5 m/s². This acceleration is responsible for increasing the car's speed as it moves around the track, and it acts along the direction of the car's velocity.
Recommended video:
Equating Accelerations
To solve the problem, we need to find the time when the magnitudes of centripetal and tangential accelerations are equal. This involves setting the expressions for both accelerations equal to each other and solving for the time variable. This requires understanding how both types of acceleration depend on the car's speed and the radius of the track.
Recommended video: