Torque on Discs & Pulleys - Online Tutor, Practice Problems & Exam Prep

Understanding torque is essential for analyzing the rotational motion of disc-like objects, such as pulleys and cylinders. The torque tau produced by a force depends on the distance (\( r \)) from the axis of rotation to the point where the force is applied, not merely the radius of the object. For example, when two masses are connected by a string around a cylinder, only the forces acting at the edges contribute to the net torque. The net torque can be calculated by summing the individual torques, considering their direction, leading to a comprehensive understanding of rotational dynamics.

Torque on Discs & Pulleys

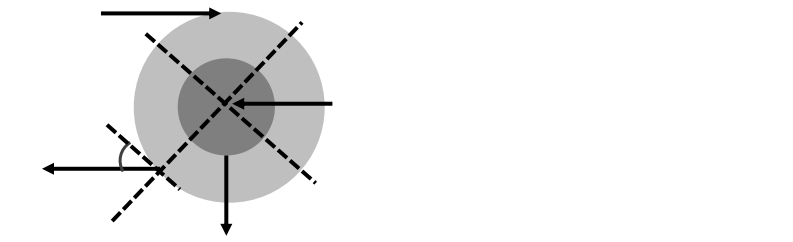
Hey, guys. So in this video, we're going to start talking about torques acting on discs, on disc-like objects such as pulleys or cylinders. This is important because it's going to be a key part of more elaborate problems we're going to have to solve later on. So let's check it out. Alright. So let's say you have a disc or a pulley, and you're pulling on it like this with the force of f, which then causes it to spin like this. Now what matters, as it says here, what matters is r, which is the distance from the axis to the force, not the radius. In this particular example here, little r is the same as the radius because the rope pulls on the disc at the edge of the disc. But let's say you were pulling with another force right here, F2. In this case, the force doesn't pull from the edge, so what matters is not the radius of the disc, but the distance, in this case, r2 is not the radius. So what always matters is the distance; most of the time, the distance will be the radius of the disc, but sometimes it won't be.

Let's do an example here. So 2 masses, m1 and m2. m1 is 4. Let's put it here. m2 is 5, are connected by a light string which passes through the edge of a solid cylinder. The cylinder has mass m3 equals 10. And radius, remember radius is big R. Little r is distance. The system is free to rotate about an axis that is perpendicular to the cylinder. To the cylinder and through its center. So basically, the cylinder spins around its central axis. We want to know what is the net torque produced on the cylinder when you release the blocks.

The net torque, torque_net, is the sum of all torques and we want to know the net torque on the cylinder. So we must figure out how many torques act on the cylinder. Remember, a force may produce a torque. First, I want to show you how there is no torque due to m3g and due to tension because they act on the axis of rotation. So the torque of t, right, the torque of any force is F r sin of Theta. In this case, F is t. So the torque of t is t r sin of Theta. But the tension is pulling. The tension is pulling from the middle here. It's holding the cylinder from the middle. So this r is 0. Now this would have been 0 even if the tension was somehow holding it up here. The problem with this part is that the tension pulls it up. Let me draw it over here. Tension let's say tension was going this way. You have to draw the r vector from the middle to the point where the force happens, the r vector. And these two arrows are both going in the same direction, which means that the angle between them is 0 degrees, which means that here you would plug in sine of 0, which is 0. So whether the tension pulls in the middle or if it pushes in the edge, it doesn't matter. They make the same. They have the same angle with each other here. So this whole thing would be 0. The torque due to mg is for sure in the middle. So it's mg0, and it also makes an angle of 0 degrees. So both of these factors don't actually produce any torque. So the only forces that would produce a torque are m1 and m2. The only forces that cause a torque are forces that could cause it to potentially spin, and that's what you get with m1 that's trying to do this to the disc, and m2 that's trying to do this. So torque 1 and torque 2.

So if you imagine a disc, if you pull from the edge of the disc, it would roll from either side. Cool. So let's now calculate the torque due to these two forces. So I'm going to call this Torque₁, which is force, which is m1g r sin of theta. So what's the r vector for m1g? Well, it's acting m1 is acting all the way at the edge of the disk because m1, the cable for m1 is passing through the outside, the edge of the disk. So the r vector is exactly the radius. So r1 is the radius. And by the way, that's the same thing that happens with m2g. R2 is also the radius because both of these guys are all the way at the edges. The angle between these guys, both of them is 90 degrees. So look how the r vector and the force make an angle of 90, and the r vector over here and its respective force makes an angle of 90 degrees. K? So both of these guys, we're going to have that the distance is the entire radius. Boom. Boom. And the angle is 90 degrees. So this obviously becomes a 1. And now I just have to multiply the numbers. The last thing you got to do is also figure out the direction. Is it positive or negative, the direction of the rotation? The M1g is trying to do this. This is if you do a complete sort of spin with your hand, you see that this is counterclockwise, so it's positive. This one is in the direction of the clock, so it's clockwise, so it's negative. Alright? So torque₁ is going to be positive. M1 is 4. G, we're going to round to 10, and the radius of this thing is 3. So this is going to be 120 Newton meter. And then for Torque₂, negative, the mass is 5, gravity rounding to 10, and the radius is 3 meters. So this is going to be negative 150 Newton meter. And when you add the whole thing, the sum of all torques will be 120 positive plus 150 negative. So the net torque is going to be negative 30 Newton meter. And that's it for this one. So hopefully, this made sense. Let me know if you have any questions. Let's keep going.

Net Torque on a disc

Hey, guys. So in this video, I want to show you how to calculate torque on a disc with forces in a bunch of different directions. Now, this picture looks sort of scary at first. What's going on with all these arrows? And you may not see something as complicated as this. I'm putting it all together so we can talk about it all at once, but you should know how to handle all these different situations individually or together. Let's check it out.

So here we have a composite disc, which means there are 2 different discs. You got the inner disc, which is the dark one here, and then the outer disc here. This allows you to have 2 different radii. They are free to rotate about a fixed axis perpendicular through their center. So basically, the discs can spin this way, right? All the forces listed here are 100 newtons, and there are 4 of them. So let's just write f1, actually, let's make this one f1, f1, f2, f3, and this one is going to be f4. They are all 100, and the angles are 37. The only angle here is 37; the rest are either flat in the x or y. The dotted lines are either exactly parallel or exactly perpendicular to each other. What does that mean? That just means that this line is the same as this line. They're parallel to each other, and these lines here make a 90-degree angle. So all these lines are making 90-degree angles with each other. Cool.

The inner disc has a radius of 3 meters. What that means is that this distance here is 3 meters, and the outer disc has a radius of 5 meters, which means that this entire distance over here is 5 meters. Okay?

We want to know the net torque produced on the disc about its central axis, and we're going to use plus or minus to indicate direction, clockwise, counterclockwise. So, the net torque is the same thing as the sum of all torques. There are 4 forces, so there are potentially 4 torques. Remember, a force may cause a torque. There are 4 forces. You could have as many as 4 torques, but some forces may not cause a torque.

Let's do one by one here. Torque 1, this guy is f1. Let's first leave a space for the sign, positive or negative, so we don't forget. f1rone sine ofθ1. And we'll calculate the sine a little later. The first thing we do is we draw an r vector, then we figure out θ, and then we work out the sine. Okay? So the r vector, is the distance, is the vector from the axis of rotation to the point where the force happens. In this case, the r vector for f1 is an arrow this way. This is r1. And r1 has a length of the outer disc, which is 5. Okay. And sine of θ. The angle that r1 makes with f1 is 90 degrees. So I'm going to put 90 here. Now in terms of sine, imagine you have a disc, and you're pushing this way on the disc. So you have a disc, and you're pulling like this, so the disc is going like this because you're pulling this direction. Right? You can also imagine as you're sort of stroking the disc this way, right? The disc is going to spin like that. So this is going to be a positive torque because it's causing the disc to spin in a counterclockwise direction of the unit circle. So it's positive. This is 1, and you just end up with positive 500 Newton meter. Alright.

So, torque 2: box f2r2sine of θ2. I know f2 is 100, but I got to figure out r and θ. So I'll leave those blank. F2 is right here, f2 acts in the middle of, acts on top of the axis of rotation. Therefore, the r2 will be 0. R2 equals 0, which means there is no torque at all. When you have something that pulls on the axis of rotation, it produces no torque because there is no r. And you can see from the equation that the whole thing becomes 0. So it doesn't matter what the angle is, and it doesn't matter what the sign is because you just have 0.

For torque 3, again, leave space for positive or negative f3r3sine ofθ3 and the force is 100. We got to figure out r and θ. If you look at f3, f3 acts on the edge of the outer disk. This is what the r3 vector looks like. Right. R3 right here. So r3 vector has a length of the outer radius, which is 5. But the problem is these two arrows make an angle of 180 degrees with each other, and the sine of 180 is 0. Okay. Sine of 180 is 0. So it doesn't matter what the sign is because, whether it's positive or negative, the direction, because the torque will be 0. Imagine the disc. And if you push directly towards the middle of the disc, you don't cause the disc to spin. The only way to cause the disc to spin is to either push sort of tangentially on the disc or to push at an angle. Right? So if you have a disc and you go like this, it's going to spin. But if you push like this on a disc, it doesn't spin.

Now let's do torque 4. This is the ugly one up here, and let's figure out what happened. So, box, I'm just going to jump straight into it. The f4 is a 100 radius sine ofθ4. So this one, we will slow down a little bit and be a little more careful. Notice that it's touching on the inner radius, the inner disc. So I'm just going to redraw just the inner disc. I'm going to write here that this has a radius of 3 because it's the inner one. Let me make this a little bigger. Radius equals 3. This force acts like this right there. This is the center. First thing we draw is the axis, the r vector. The r vector is from the axis to the point where the force happens. Notice that here, this would look like this. So this dotted line is just an extension of your R vector. And there's an angle of 37 degrees here. Okay. So you got to figure out which angle to use. First of all, the distance will be the entire radius of the inner circle of the inner disc. So it's 3. And what about the vector? So what about the angle? So what you could do is you get the r vector here, and you can extend it this way. Right? And to make it easier to notice that this is, in fact, the angle you should use. It's the angle between the two lines. So 37 is the correct angle. Okay. Remember, the angle given to you isn't always the one you're supposed to use. In fact, it's usually not the one you're supposed to use. But in this case, it turned out to be that way. What about the direction in which this thing will spin? So you should imagine that if you're pushing a disc like this, it's actually going to spin like this. One way that would make this easier is to think of this as a not as a push on the disc, but as a pull. You're essentially pulling the disc. I'm sort of redrawing this f4 over here, just kind of extending it down. You're pulling, you're pushing this way. You're causing it to go like that. Okay. So hopefully that makes sense. It's pretty visual, but hopefully, you can follow that. So this direction here is in the direction of the unit circle. It's counterclockwise, so it's positive. Okay. Positive. And if you multiply this whole thing, you get that torque 4 is positive 180 Newton meter.

To find the net torque, we just add everything up. I got 2 of them that were 0. So it's just the positive 500 and the positive 180, which gives you a positive 680 Newton meter. Okay? That's it for this one. Hopefully, it makes sense. Let me know if you have any questions, and let's keep going.

The composite disc below is free to rotate about a fixed axis, perpendicular to it and through its center. All forces are 100 N, and all angles are 37°. The dotted lines are either exactly parallel or exactly perpendicular to each other. The inner (darker) and outer (lighter) discs have radii 3 m and 5 m, respectively. Calculate the Net Torque produced on the composite disc, about an axis perpendicular to it and through its center. Use +/– to indicate direction.

• A. −100 N•m
• B. +100 N•m
• C. −800 N•m
• D. +800 N•m
• E. −900 N•m
• F. +900 N•m
• G. −1440 N•m
• H. +1440 N•m

A square with sides 4 m long is free to rotate around an axis perpendicular to its face and through its center. All forces shown are 100 N and act simultaneously on the square. The angle shown is 30°. Calculate the Net Torque that the forces produce on the square, about its axis of rotation.

• A. +207 N•m
• B. +327 N•m
• C. +348 N•m
• D. +473 N•m

Here’s what students ask on this topic:

What is the formula for calculating torque on a disc or pulley?

The formula for calculating torque (τ) on a disc or pulley is given by:

$\tau =Fr\sin \left(\theta \right)$

where:

• $F$ is the force applied.
• $r$ is the distance from the axis of rotation to the point where the force is applied.
• $\theta$ is the angle between the force vector and the lever arm.

This formula helps in understanding how different forces and their points of application affect the rotational motion of the disc or pulley.

How do you determine the net torque on a pulley system with multiple forces?

To determine the net torque on a pulley system with multiple forces, follow these steps:

1. Identify all the forces acting on the pulley.
2. Calculate the torque produced by each force using the formula $\tau =Fr\sin \left(\theta \right)$.
3. Determine the direction of each torque (clockwise or counterclockwise).
4. Sum the torques, taking into account their directions (positive for counterclockwise, negative for clockwise).

The net torque is the algebraic sum of all individual torques. This helps in understanding the overall rotational effect on the pulley.

Why does the weight of the pulley not contribute to the net torque?

The weight of the pulley does not contribute to the net torque because it acts through the axis of rotation. Torque is given by $\tau =Fr\sin \left(\theta \right)$, and when the force (weight) acts at the axis, the distance $r$ is zero. Therefore, the torque produced is zero. Additionally, forces that act along the axis of rotation do not create a lever arm, resulting in no rotational effect.

How do you calculate the torque produced by a force acting at the edge of a disc?

To calculate the torque produced by a force acting at the edge of a disc, use the formula:

$\tau =Fr\sin \left(\theta \right)$

where:

• $F$ is the force applied.
• $r$ is the radius of the disc (since the force is applied at the edge).
• $\theta$ is the angle between the force vector and the radius (usually 90 degrees, making $\sin \left(90\right)=1$).

This simplifies to $\tau =Fr$ when the force is perpendicular to the radius.

What factors affect the magnitude of torque on a disc or pulley?

The magnitude of torque on a disc or pulley is affected by three main factors:

• Force (F): The greater the force applied, the larger the torque.
• Distance (r): The distance from the axis of rotation to the point where the force is applied. A larger distance results in a greater torque.
• Angle (θ): The angle between the force vector and the lever arm. Torque is maximized when the angle is 90 degrees, as $\sin \left(90\right)=1$.

These factors combine in the formula $\tau =Fr\sin \left(\theta \right)$ to determine the overall torque.