Intro to Moment of Inertia - Online Tutor, Practice Problems & Exam Prep

The moment of inertia (I) is the rotational equivalent of mass, representing an object's resistance to changes in its rotational velocity. It depends on both mass and the distance from the axis of rotation. For point masses, the moment of inertia is calculated using the equation $m^{2}r$, while for rigid bodies, it varies based on shape and is typically found in reference tables. The units for moment of inertia are kilogram meters squared (kg·m²), indicating the resistance to angular acceleration.

Intro to Moment of Inertia

Hey, everyone. So in this video, we're gonna talk about something called the moment of inertia, which you can think of as kind of like the rotational equivalent of mass. Remember that when we talked about linear motion, mass was the quantity of an object's resistance to changes in velocity. Well, similarly, what we're gonna see is that the moment of inertia is the same idea but for rotational motion. It's the amount of resistance that something has to changes in its rotational velocity. Alright? So I want to talk about the differences between these 2, and then we'll do a couple of examples together. So let's check it out.

Before we start, I just want to recap everything we've talked about with linear motion. Remember that when we talked about linear motion and did linear motion problems, we used our 3 to 4 equations of motion, and they did not depend on the mass. Right? We didn't have mass anywhere in those motion equations. Then when we started talking about energy and forces, mass was important. Right? We have 12mv² and then we also have F = ma. So similarly, before we start talking about rotational kinetic energy and rotational forces, we're gonna have to talk about what the equivalent of mass is for rotation, and that's what this moment of inertia is.

Remember, with linear motion, mass was the amount of resistance to linear acceleration. Right? If you have a higher mass, you have a higher resistance to changes in velocity. We call that property, that resistance to change, inertia. Remember, inertia just means that you want to keep doing whatever it is that you were doing before. If you're at rest, you stay at rest. If you're in motion, you stay in motion. That's inertia. We can actually see this directly from F = ma. Right? If mass is m, then if we have F = ma and you rearrange for acceleration, acceleration is just force divided by mass. So we can see here that if your mass increases, then your acceleration decreases. Right? If you have higher mass, your change in velocity is lower. And so we call that that resistance, that was basically what mass was. So a higher resistance just means that you have a higher inertia.

In rotation, what happens is that the moment of inertia is the amount of resistance to angular acceleration. With linear motion, mass was resistance to linear acceleration. With rotation, the moment of inertia is resistance to angular acceleration. It depends on 2 things, it depends on mass and also the distance to the axis. With linear motion, it only depended on mass, but with rotational motion, the moment of inertia depends on mass and also how far you are from the axis of rotation.

So I want you to picture a yo-yo that's sort of going around like this, on a string. And if it's at 10 centimeters versus 20 centimeters, this one has lower inertia, but at 20 centimeters because you're doing a bigger circle, you have a higher resistance to rotational motion. This combination of mass and distance to the axis is called the moment of inertia. Both of those things make up the moment of inertia. Now that moment of inertia takes the letter I, so you can think of I for inertia, and it's the rotational equivalent of mass as we've said before. You can think of it as rotational inertia. It's the resistance to change in angular or rotational velocity.

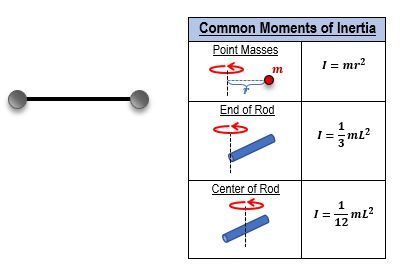
There are 2 kinds of objects that we're going to cover in your problems. You're going to see point masses and also rigid bodies or other shapes. For a point mass, it would be kind of like a yo-yo or a ball or marble on a string that goes around in a circle. So imagine that you have a little string like this and you've got a little point mass here that's on the end and it's going around in a circle. The radius of this circle that you're making is little r. For a point mass because it's so small, a lot of times your problems will say it's a very small object or something like that. We can say that its radius is equal to 0. This little r is a distance, but this big R is a radius. We're going to treat these objects as if they're really tiny; they don't have a lot of volume.

On the other hand, you might see something called a rigid body or a shape something like a cylinder or a sphere, and rigid bodies do not have zero radius. They actually do have some size. So a very common one that you're going to see is a cylinder like this. You have a cylinder like that, and you'll have an axis of rotation that goes through, and you're going to try to spin the cylinder on its axis. This object does have a radius because it does have finite size, and that's going to be the big R, okay? That's the difference between those 2. In one, you have a distance little r and in one you actually have a radius.

So let's talk about the moments of inertia for these 2. For a point mass, the moment of inertia equation is actually very straightforward. It's just m times little r squared, and again that little r is just going to be the distance to the axis of rotation. For rigid bodies or shapes, if you have a cylinder or a sphere, it's going to be different. You're going to find this moment of inertia by looking it up in a table. Your textbook will have a table. You'll see some pretty shapes and they'll have different formulas for the moment of inertia. But one thing I want to mention here is that the general format for a moment of inertia is going to be some kind of a fraction times mr². So you'll see that for point masses it's mr² and for something like spinning a rod through the center, you would have some distance squared.

Let's just go ahead and get into our problems here. We have a system of point masses that are basically on the ends of this rope. We want to calculate the moment of inertia of the system if it spins about the perpendicular axis through the center of the rod. So we're going to calculate the moment of inertia of the system. In other words, we're going to calculate I_system. That's really what we're looking for here.

The axis, if it spins about a perpendicular axis through the center of the rod. So this is the rod like this. We want perpendicular, remember that just means 90 degrees. There are 2 ways you could do this. If the rod is like this, you could have this as my axis or you could also have this going back and forth as your axis. So obviously, it's going to be this because it's going to spin around sort of horizontally like that. So you can kind of imagine that it's going to spin like this, right? Sort of on the same plane as you. That's how it's going to spin, through the center of the rod as shown.

How do we calculate this moment of inertia? Well, in general, the moment of inertia of a system of objects is just going to be the sum of each moment of inertia of the objects that make it up. What do I mean by this? In this problem, we have a rod, and by the way, the rod is massless. So what we have here is that m_rod = 0, but we also have these two point masses on the end. I'm going to call this m_r, because it's 4 kilograms, and this one's going to be m_l, which is 3. Both of these pieces, these little point masses here, are some distance away from the axis of rotation. And what we said here is that for point masses, there is a moment of inertia, m_r. So we actually have 2 of them in this problem. And we also have a rod that is also spinning. Everything is spinning in this problem, the

A system is made of two small masses (M_LEFT = 3 kg, M_RIGHT = 4 kg) attached to the ends of a 5 kg, 2-m long thin rod, as shown. Calculate the moment of inertia of the system if it spins about a perpendicular axis through the mass on the left.

• A. 8.67 kg•m²
• B. 15.7 kg•m²
• C. 17.7 kg•m²
• D. 22.7 kg•m²

Moment of inertia of Earth

Hey, guys. So, as you know, the Earth is rotating. Therefore, it has a moment of inertia. And if we make some assumptions about the shape of the Earth, we can actually calculate the moment of inertia of the Earth. Let's check it out. It says here the Earth has a mass and radius given by these big numbers. And then I also tell you that the radial distance between the Earth and the Sun is this. What I mean by radial distance is that, if the Sun is here, the Earth spins around the Sun at this distance here; little r is \(1.5 \times 10^{11}\). Squeeze it in there. Cool? That's what I mean by that. And then I gave you the mass of the Earth and the radius of the Earth as well. I want to know the moment of inertia of the Earth as it spins around itself and as it spins around the Sun. As you know, the Earth has 2 oceans, and we can calculate a moment of inertia about, or relative to those 2 motions or for those 2 motions. Remember, moment of inertia depends on the axis of rotation. That's why these numbers will be different. So if you want to know the moment of inertia of the Earth around itself, you would have to treat the Earth as an object with significant size. You can't treat it as a tiny object. So what we do here is we're going to treat the Earth as a solid sphere. Okay? As a solid sphere. So the Earth is a big ball that spins around itself. Now technically, it's at an angle like that, but it doesn't really matter. You can just do this. Okay? So it's spinning around itself. And your book would show you that solid spheres have a moment of inertia given by this equation right here. So when I tell you solid sphere, I'm indirectly telling you, hey, use this equation for I. Okay? So for part a, we're going to do, part a is over here, we're going to say \(I = \frac{2}{5}mr^2\). And all we've got to do is plug in the numbers here. So m is the mass of the Earth, which is \(5.97 \times 10^{24}\), and r is the radius of the Earth, which is this, and not the radial distance. It's the Earth going around itself, so it's the radius of the actual object of the sphere. \(6.37 \times 10^6\)^2. Okay. If you look at this number, I got a 24, and then I got a 6 squared. So you should imagine that this is going to be a gigantic number, and it is. I multiplied everything. I get \(9.7 \times 10^{37}\) kilograms meter square. The Earth has a lot of inertia. And what that means is that it would be incredibly hard to make the Earth stop spinning. Okay? Now if you were to Google this number, you would see that it's actually a little bit off. The actual moment of inertia is a little bit off. And that's because the Earth is not a perfectly, a perfect sphere. It's got different layers. It's not even a sphere. So but this number is a pretty good approximation. For part b, we want to find out what is the moment of inertia of the Earth as it spins around the Sun. Now in this case, relative to the sun, the Earth is tiny. So we're going to treat it as a point mass, which is crazy. The Earth is a huge thing, and you're going to just treat it as a little point mass of negligible radius. And that's because, relative to the sun, the Earth is negligible in size. Okay? So I'm going to put the Earth here as a tiny m, earth, and it's going around the sun. And the distance here, the radial distance, which is little r, big r is the radius of an object, and little r is the distance to the center, is \(1.5 \times 10^{11}\) meters. In this case, we're going to use, instead of \(\frac{2}{5}mr\), we're going to use \(mr^2\) because the Earth is being treated as a point mass here. M is the mass of the object itself. Right? So it's the object that's spinning. It's not the sun. So it's going to be \(5.97 \times 10^{24}\), the same thing. But r is going to be the distance to the center, which is 1.5. So \(1.5 \times 10^{11}\)^2. I got a 24. I got an 11 squared. This is going to be, again, a gigantic number, \(1.34 \times 10^{47}\). This number is, like, a billion times bigger than the other number. Right? So as hard as it would be to stop the Earth from stopping, to get the Earth to stop spinning, it would be way harder. Right? It would be \(10^{10}\) times harder to make the Earth stop going around the Sun. And that's it. So that's it for this one. It's a very typical, classic problem. Hopefully, it makes sense. You should try this out on your own and let's keep going.

A solid disc 4 m in diameter has a moment of inertia equal to 30 kg m² about an axis through the disc, perpendicular to its face. The disc spins at a constant 120 RPM. Calculate the mass of the disc.

• A. 1.88 kg
• B. 3.75 kg
• C. 7.5 kg
• D. 15 kg

Inertia of planet of known density

Hey, guys. So here's another pretty straightforward moment of inertia question. The only difference here is that we're going to have density to deal with. So let's do this real quick. Since we have a planet that is nearly spherical, nearly spherical means that it's a sphere. Okay. So you can basically ignore the word nearly. It means we're going to approximate it as a sphere with nearly continuous mass distribution. Again, you can ignore the word nearly and assume that it has continuous mass distribution. Continuous mass distribution, we'll talk more about this later, but it basically means that the sphere has the mass evenly distributed throughout the sphere. So sometimes you see drawn like something like this. This is a solid sphere and this is opposed to a hollow sphere, which is a sphere that has nothing inside. It's not continuously or evenly distributed. All the mass is concentrated on the edges. This is not what we have here. This is what we have here. The reason why that's important is because you're going to get a different I equation, a different moment of inertia equation depending on what kind of sphere you have. And the moment of inertia equation for this guy here is:

2 5 m r 2

The question didn't give you this, but you would look this up in your book or in a test. The professor would have to give you this somehow, unless your professor requires you to memorize this, then you'd have to do that. But most of them don't. Alright? So that's the equation you're supposed to use. I give you the radius right here. Radius is \(8 \times 10^7\) meters and I give you the density. Density, you can use d, but the official variable, if you will, is rho. Right? It's \(10,000 \, \text{kg/m}^3\). I want to remind you that if you have a volume, the density of a volume is mass over volume, and you could have seen this from the units, kilograms per cubic meter. Right? So it's a volume. It's a 3-dimensional object and that's it. That's all we're given. I also give you here the equation for the volume of a sphere. Volume right here of a sphere. Now if you were looking for I and if you start putting stuff in here, you would realize you don't have m, but you have r. Okay. So we don't have m. We gotta figure this out. And if you look around, you realize, well, I have another piece of information that has some connection to m. So maybe I can use this to solve for m and that's exactly what we're supposed to do. So I want to find m. I have 10,000, but I don't have v. But once again, I have another piece of information here that allows me to find v. V equals, the volume of a sphere is \(4/3 \pi r^3\) and I know r. I know r, so I can get v. I'm going to know v, so I'm going to be able to get m. I'm going to know m, so I'm going to be able to get I. Okay. That's how it's going to flow. Alright. So what I'm going to do is right here, I'm going to solve for m. In other words, I'm going to move v over here. So m equals 10,000v and v is according to this equation right here, \(4/3 \pi r^3\). So I'm going to get this whole thing, which is m and I'm going to stake it in here. Okay. So

2 5 10,000 4 3 π r 3 r 2

These two terms combined \( r^5 \). So it's going to be \( 8 \times 10^7 \) to the 5th. Okay, so you should expect a pretty big number and I get \(5.49 \times 10^{43}\). Now, how exactly you arrive at this number doesn't really matter. It's I, so it's kilograms meter squared. You could have, you know, gotten a number here, plugged in here. It really doesn't matter as long as you arrive here. It's a bunch of multiplication. Cool. So that's it for this one. Let me know if you have any questions.

Here’s what students ask on this topic:

What is the moment of inertia and how is it related to mass?

The moment of inertia (I) is the rotational equivalent of mass, representing an object's resistance to changes in its rotational velocity. It depends on both the mass of the object and the distance from the axis of rotation. For linear motion, mass is the resistance to linear acceleration, while for rotational motion, the moment of inertia is the resistance to angular acceleration. The formula for a point mass is given by $I_{\mathrm{point}}=m{r}^2$, where m is the mass and r is the distance to the axis of rotation.

How do you calculate the moment of inertia for different shapes?

The moment of inertia for different shapes varies and is typically found in reference tables. For point masses, it is calculated using $I_{\mathrm{point}}=m{r}^2$. For rigid bodies, the formula depends on the shape and axis of rotation. For example, the moment of inertia of a solid cylinder rotating about its central axis is $I_{\mathrm{cylinder}}=\frac{1}{2}m{r}^2$, where m is the mass and r is the radius.

What are the units of moment of inertia?

The units of moment of inertia are kilogram meters squared (kg·m²). This unit indicates the resistance to angular acceleration. The formula for moment of inertia involves mass (in kilograms) and the square of the distance to the axis of rotation (in meters), resulting in the combined unit of kg·m².

Why does the moment of inertia depend on the distance to the axis of rotation?

The moment of inertia depends on the distance to the axis of rotation because it measures how mass is distributed relative to the axis. The farther the mass is from the axis, the greater the resistance to rotational motion. This is why the formula for a point mass includes the term $r^2$. For example, a mass at a larger radius from the axis will have a higher moment of inertia compared to the same mass closer to the axis.

How does the moment of inertia affect rotational motion?

The moment of inertia affects rotational motion by determining how much torque is needed to achieve a certain angular acceleration. A higher moment of inertia means more resistance to changes in rotational velocity, requiring more torque to accelerate or decelerate the object. This is analogous to how mass affects linear motion, where a higher mass requires more force to change its velocity.