Here are the essential concepts you must grasp in order to answer the question correctly.
Osmotic Pressure
Osmotic pressure is the pressure required to prevent the flow of solvent into a solution through a semipermeable membrane. It is directly proportional to the concentration of solute particles in the solution. The formula for osmotic pressure (π) is π = iCRT, where 'i' is the van 't Hoff factor, 'C' is the molar concentration, 'R' is the ideal gas constant, and 'T' is the temperature in Kelvin.
Recommended video:
Osmotic Pressure Concept 1
Van 't Hoff Factor (i)
The van 't Hoff factor (i) indicates the number of particles into which a solute dissociates in solution. For example, NaCl dissociates into two ions (Na+ and Cl-), so its van 't Hoff factor is 2. In contrast, glucose does not dissociate in solution, giving it a van 't Hoff factor of 1. This factor is crucial for calculating osmotic pressure accurately.
Recommended video:
Conversion Factors (Simplified) Concept 1
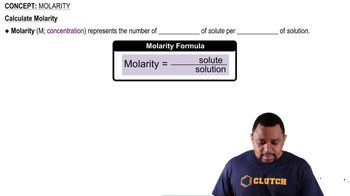
Molarity and Concentration
Molarity is a measure of concentration defined as the number of moles of solute per liter of solution. To compare osmotic pressures, it is essential to calculate the molarity of each solution. The total number of solute particles, influenced by both the mass of solute and its molar mass, determines the osmotic pressure, making concentration a key factor in this analysis.
Recommended video:
 Verified step by step guidance
Verified step by step guidance