A 20.0-L volume of an ideal gas in a cylinder with a piston is at a pressure of 3.0 atm. Enough weight is suddenly removed from the piston to lower the external pressure to 1.5 atm. The gas then expands at constant temperature until its pressure is 1.5 atm. Find w.
The ΔH°f of TiI3(s) is –328 kJ/mol and the ΔH ° for the reaction 2 Ti(s) + 3 I2(g) → 2 TiI3(s) is –839 kJ. Calculate the ΔH of sublimation of I2(s), which is a solid at 25 °C.
 Verified step by step guidance
Verified step by step guidance
Verified Solution
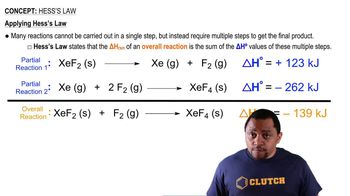
Key Concepts
Enthalpy of Formation (ΔH°f)

Sublimation

Hess's Law
When 10.00 g of phosphorus is burned in O2(g) to form P4O10(s), enough heat is generated to raise the temperature of 2950 g of water from 18.0 °C to 38.0 °C. Calculate the enthalpy of formation of P4O10(s) under these conditions.
The ΔH for the oxidation of sulfur in the gas phase to SO3 is –204 kJ/mol and for the oxidation of SO2 to SO3 is 89.5 kJ/mol. Find the enthalpy of formation of SO2 under these conditions.
A gaseous fuel mixture contains 25.3% methane (CH4), 38.2% ethane (C2H6), and the rest propane (C3H8) by volume. When the fuel mixture contained in a 1.55 L tank, stored at 755 mmHg and 298 K, undergoes complete combustion, how much heat is emitted? (Assume that the water produced by the combustion is in the gaseous state.)
A gaseous fuel mixture stored at 745 mmHg and 298 K contains only methane (CH4) and propane (C3H8). When 11.7 L of this fuel mixture is burned, it produces 769 kJ of heat. What is the mole fraction of methane in the mixture? (Assume that the water produced by the combustion is in the gaseous state.)
