Explain the observed trend in the boiling points of these compounds.
H2Te -2 °C
H2Se -41.5 °C
H2S -60.7 °C
H2O 100 °C
 Verified step by step guidance
Verified step by step guidance
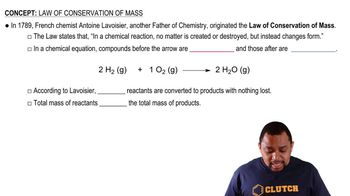

Explain the observed trend in the boiling points of these compounds.
H2Te -2 °C
H2Se -41.5 °C
H2S -60.7 °C
H2O 100 °C
The vapor pressure of water at 25 °C is 23.76 torr. If 0.25 g of water is enclosed in a 1.50-L container, will any liquid be present? If so, what mass of liquid?
The vapor pressure of CCl3F at 300 K is 856 torr. If 3.5 g of CCl3F is enclosed in a 1.0-L container, will any liquid be present? If so, what mass of liquid?
Air conditioners not only cool air, but dry it as well. A room in a home measures 6.0 m × 10.0 m × 2.2 m. If the outdoor temperature is 30 °C and the partial pressure of water in the air is 85% of the vapor pressure of water at this temperature, what mass of water must be removed from the air each time the volume of air in the room is cycled through the air conditioner? (Assume that all of the water must be removed from the air.) The vapor pressure for water at 30 °C is 31.8 torr.
A sealed flask contains 0.55 g of water at 28 °C. The vapor pressure of water at this temperature is 28.35 mmHg. What is the minimum volume of the flask in order that no liquid water be present in the flask?
Based on the phase diagram of CO2 shown in Figure 11.39(b), describe the state changes that occur when the temperature of CO2 is increased from 190 K to 350 K at a constant pressure of (a) 1 atm