A 1.0-L container of liquid nitrogen is kept in a closet measuring 1.0 m by 1.0 m by 2.0 m. Assuming that the container is completely full, that the temperature is 25.0 °C, and that the atmospheric pressure is 1.0 atm, calculate the percent (by volume) of air that is displaced if all of the liquid nitrogen evaporates. (Liquid nitrogen has a density of 0.807 g/mL.)
Aerosol cans carry clear warnings against incineration because of the high pressures that can develop upon heating. Suppose that a can contains a residual amount of gas at a pressure of 755 mmHg and a temperature of 25 °C. What would the pressure be if the can were heated to 1155 °C?

Verified Solution
Key Concepts
Gas Laws

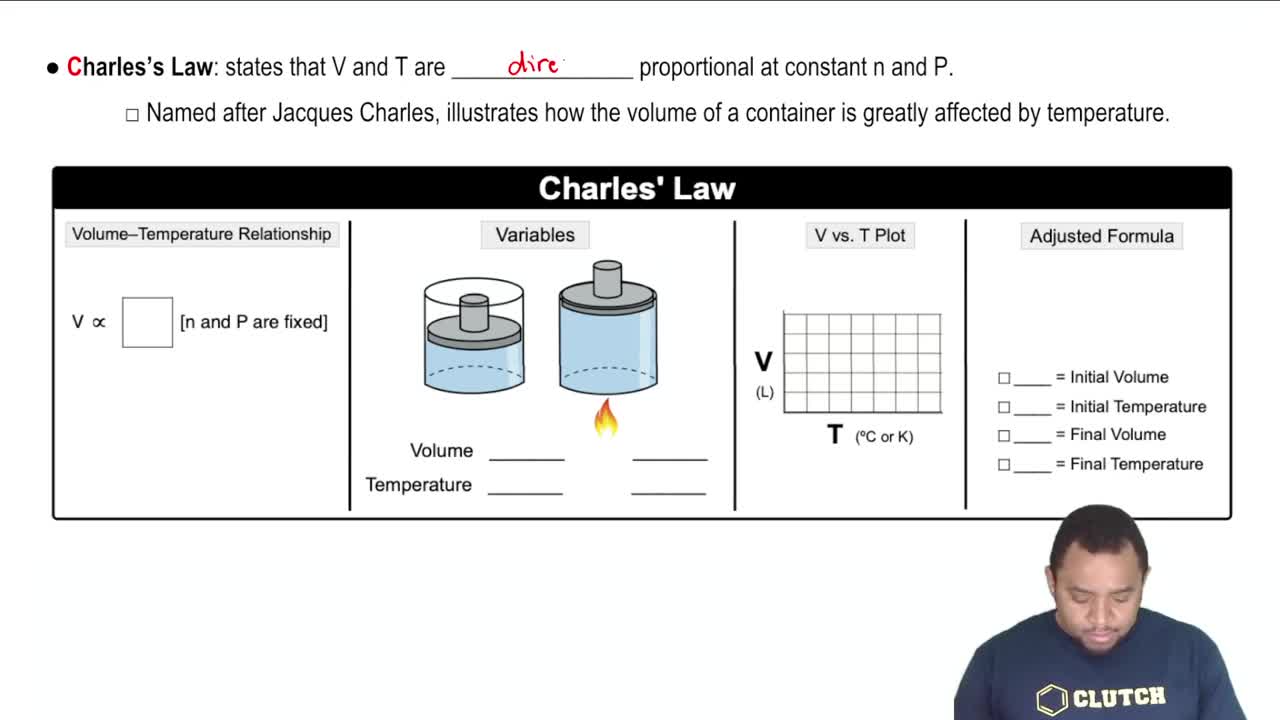
Charles's Law
Absolute Temperature

Which gas sample has the greatest pressure? Assume that all the samples are at the same temperature. Explain.
This picture represents a sample of gas at a pressure of 1 atm, a volume of 1 L, and a temperature of 25 °C. Draw a similar picture showing what would happen to the sample if the volume were reduced to 0.5 L and the temperature were increased to 250 °C. What would happen to the pressure?
A sample of nitrogen gas in a 1.75-L container exerts a pressure of 1.35 atm at 25 °C. What is the pressure if the volume of the container is maintained constant and the temperature is raised to 355 °C?
Use the molar volume of a gas at STP to determine the volume (in L) occupied by 33.6 g of neon at STP.
Use the molar volume of a gas at STP to calculate the density (in g/L) of nitrogen gas at STP.
