According to Coulomb's law, rank the interactions between charged particles from lowest potential energy to highest potential energy. a. a 1+ charge and a 1- charge separated by 100 pm b. a 2+ charge and a 1- charge separated by 100 pm c. a 1+ charge and a 1+ charge separated by 100 pm d. a 1+ charge and a 1- charge separated by 200 pm
If core electrons completely shielded valence electrons from nuclear charge (i.e., if each core electron reduced nuclear charge by 1 unit) and if valence electrons did not shield one another from nuclear charge at all, what would be the effective nuclear charge experienced by the valence electrons of each atom? a. K
 Verified step by step guidance
Verified step by step guidance
Verified Solution
Key Concepts
Effective Nuclear Charge (Z_eff)

Shielding Effect

Valence Electrons
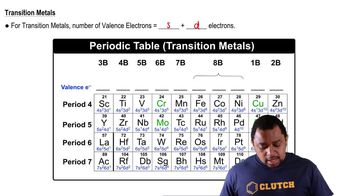
Which experience a greater effective nuclear charge: the valence electrons in beryllium or the valence electrons in nitrogen? Why?
Arrange the atoms according to decreasing effective nuclear charge experienced by their valence electrons: S, Mg, Al, Si.
If core electrons completely shielded valence electrons from nuclear charge (i.e., if each core electron reduced nuclear charge by 1 unit) and if valence electrons did not shield one another from nuclear charge at all, what would be the effective nuclear charge experienced by the valence electrons of each atom? b. Ca
If core electrons completely shielded valence electrons from nuclear charge (i.e., if each core electron reduced nuclear charge by 1 unit) and if valence electrons did not shield one another from nuclear charge at all, what would be the effective nuclear charge experienced by the valence electrons of each atom? c. O d. C
In Section 3.6, we estimated the effective nuclear charge on beryllium's valence electrons to be slightly greater than 2+. What would a similar treatment predict for the effective nuclear charge on boron's valence electrons? Would you expect the effective nuclear charge to be different for boron's 2s electrons compared to its 2p electron? In what way? (Hint: Consider the shape of the 2p orbital compared to that of the 2s orbital.)
