During photosynthesis, plants use energy from sunlight to form glucose (C6H12O6) and oxygen from carbon dioxide and water. Write a balanced equation for photosynthesis.
The explosive nitroglycerin (C3H5N3O9) decomposes rapidly upon ignition or sudden impact according to the balanced equation: 4 C3H5N3O9(l) → 12 CO2(g) + 10 H2O(g) + 6 N2(g) + O2(g) ΔH°rxn = –5678 kJ Calculate the standard enthalpy of formation (ΔH°f ) for nitroglycerin.

Verified Solution
Key Concepts
Standard Enthalpy of Formation (ΔH°f)

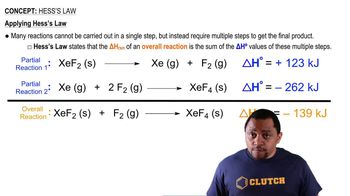
Hess's Law
Balanced Chemical Equations

Ethanol (C2H5OH) can be made from the fermentation of crops and has been used as a fuel additive to gasoline. Write a balanced equation for the combustion of ethanol and calculate ΔH°rxn.
Top fuel dragsters and funny cars burn nitromethane as fuel according to the balanced combustion equation: 2 CH3NO2(l) + 3/2O2(g) → 2 CO2(g) + 3 H2O(l) + N2(g) ΔH°rxn = –1418 kJ The enthalpy of combustion for nitromethane is –709.2 kJ/mol. Calculate the standard enthalpy of formation (ΔH°f ) for nitromethane.
Determine the mass of CO2 produced by burning enough of each fuel to produce 1.00×102 kJ of heat. a. CH4(g) + 2 O2(g) → CO2(g) + 2 H2O(g) ΔH°rxn = –802.3 kJ
Methanol (CH3OH) has been suggested as a fuel to replace gasoline. Find ΔH°rxn, and determine the mass of carbon dioxide emitted per kJ of heat produced. Use the information from the previous exercise to calculate the same quantity for octane, C8H18. How does methanol compare to octane with respect to global warming?
