Carbon-12 contains six protons and six neutrons. The radius of the nucleus is approximately 2.7 fm (femtometers) and the radius of the atom is approximately 70 pm (picometers). What percentage of the carbon atom's volume is occupied by the nucleus? (Assume two significant figures.)

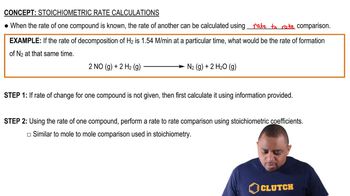
Verified step by step guidance

1. Calculate the volume of the nucleus using the formula for the volume of a sphere: $V = \frac{4}{3} \pi r^3$, where $r$ is the radius of the nucleus in femtometers.
2. Convert the radius of the atom from picometers to femtometers to ensure both radii are in the same units. (1 pm = 1000 fm)
3. Calculate the volume of the atom using the same formula for the volume of a sphere: $V = \frac{4}{3} \pi r^3$, where $r$ is the radius of the atom in femtometers.
4. Determine the percentage of the atom's volume occupied by the nucleus by dividing the volume of the nucleus by the volume of the atom and multiplying by 100%.
5. Express the final answer with two significant figures as required by the problem.

Key Concepts

Atomic Structure

The atomic structure consists of a nucleus, which contains protons and neutrons, and electrons that orbit around the nucleus. In the case of Carbon-12, the nucleus has six protons and six neutrons, while the electrons occupy a much larger volume outside the nucleus. Understanding this structure is essential for calculating the relative sizes and volumes of the nucleus and the atom.

Volume Calculation

To determine the volume of the nucleus and the atom, we use the formula for the volume of a sphere, V = (4/3)πr³, where r is the radius. The nucleus of Carbon-12 has a radius of approximately 2.7 femtometers, while the atom has a radius of about 70 picometers. Accurate volume calculations are crucial for finding the percentage of the atom's volume occupied by the nucleus.

Percentage Calculation

Calculating the percentage of the atom's volume occupied by the nucleus involves dividing the volume of the nucleus by the volume of the atom and then multiplying by 100. This concept is fundamental in comparing the relative sizes of different components within an atom, highlighting how much space the nucleus occupies in relation to the entire atom.