An ibuprofen suspension for infants contains 100 mg>5.0 mL suspension. The recommended dose is 10 mg>kg body weight. How many mL of this suspension should be given to an infant weighing 18 lb? (Assume two significant figures.)

Video transcript

All right. Hello everyone. So this question says that an Ibuprofen suspension for infants contains 100 mg per 5.0 mL of suspension. The recommended dose is 10 mg per kilogram body weight. How many, excuse me, how many milliliters of the suspension should be given to an infant weighing £18 assume two significant figures. And here we have four different answer choices, proposing different numbers or the volume of the suspension needed in milliliters. So let's go ahead and get started. Now, in this case, we're going to go ahead and use dimensional analysis to find the dose that the child needs. And then from there calculate the number of milliliters necessary from the suspension provided. But there is a slight discrepancy because notice how the child's weight is provided in pounds, but the dosage is described per kilogram of body weight. So because of that, we can see how our plan is going to proceed, right? Because first we're going to have to convert the child's weight from pounds to kilograms to use the conversion factor provided for the ideal dose of ibuprofen. So from pounds, we go to kilograms and then from kilograms, we get the ideal dose that the child needs. And so this translates to the volume needed after taking the required dose and using the data provided of the suspension to find the volume needed depending on the number of milligrams necessary for the child's dose. So before we go ahead and write everything out, recall it, 1 kg is equal to £2.205. So first I'm going to go ahead and take the child's weight in pounds, that's £18 and multiply this by the conversion factor provided to go from pounds to kilograms. Now recall that in dimensional analysis, the goal is to always eliminate or cancel your starting units. So because my starting units are pounds and my goal is to eliminate those units. I'm going to treat my conversion factor as a fraction with a numerator and a denominator. So in this case, the portion of my conversion factor that's expressed in pounds should go in the denominator, whereas kilograms should go on the numerator. This ensures that my units of pound cancel out. And my answer from the step of the calculation would be in kilograms. Now, in this case, we have a couple of different options. You can either calculate each step separately or you can place all conversion factors together and calculate everything at once. In this case, I'm going to go ahead and calculate everything at once, making sure to keep track of my units as I do. 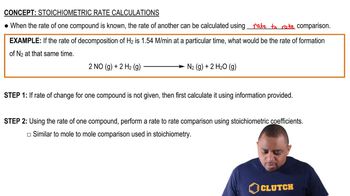So So at this point, we've used the first conversion factor to convert the child's body weight from units of pounds to units of kilograms. So at this point, we can introduce a second conversion factor defined the dose of ibuprofen that the child needs. Though in this case, the conversion factor necessary is 10 mg of ibuprofen for every kilogram of body weight. Now, because my answer is currently in units of kilograms. Kilograms should be in the denominator of my second conversion factor. So my 10 mg should go on the numerator of the second conversion factor and this ensures that kilograms cancel out. So at this point, we have just calculated the dose that the child needs, meaning that we have to find the volume necessary based on the data provided for the suspension. So our third and final conversion factor is 100 mg of Ibuprofen per every 5.0 mL of suspension. Now here because my units or rather my answer is currently in units of milligrams. Milligrams should be in the denominator of my final conversion factor which means milliliters should be in the numerator. This ensures that my units of milligrams cancel out and that my final answer is going to be in units of milliliters before I go ahead or rather after I go ahead and calculate everything at once. So in this case, our final answer is going to be determined by multiplying all of the numerators and dividing that by all of our denominators. This gives us an ungrounded answer of 4.0816 mL. However, in this case, we're going to assume two significant figures, which means that my answer would be rounded to 4.1 milliliters. And that is our final answer that matches with option D in the multiple choice, that's 4.1 milliliters and there you have it. So with that being said, if you stuck around until the end of this video, thank you so very much. I appreciate it. And I hope you found this helpful.