Platinum crystallizes with the face-centered cubic unit cell. The radius of a platinum atom is 139 pm. Calculate the edge length of the unit cell and the density of platinum in g/cm³.

Verified step by step guidance

1. insert step 1> Determine the relationship between the atomic radius and the edge length in a face-centered cubic (FCC) unit cell. In an FCC unit cell, the face diagonal is equal to four times the atomic radius (4r), and the face diagonal is also equal to the square root of 2 times the edge length (a√2).
2. insert step 2> Set up the equation for the face diagonal: 4r = a√2.
3. insert step 3> Solve for the edge length (a) using the given atomic radius (r = 139 pm): a = 4r/√2.
4. insert step 4> Convert the edge length from picometers to centimeters for the density calculation. Remember that 1 pm = 1 x 10^-12 m and 1 m = 100 cm.
5. insert step 5> Calculate the density of platinum using the formula: density = mass/volume. Use the molar mass of platinum (195.08 g/mol) and Avogadro's number (6.022 x 10^23 atoms/mol) to find the mass of one unit cell, and use the edge length to find the volume of the unit cell.

Key Concepts

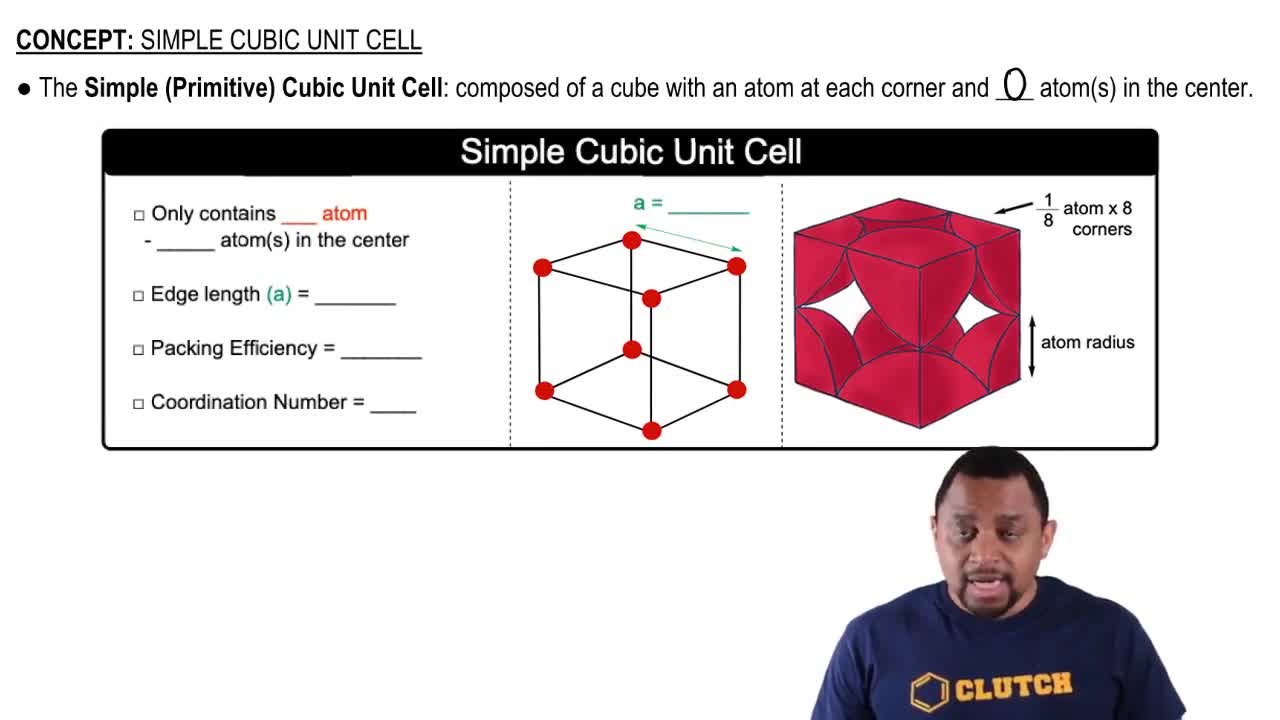
Face-Centered Cubic (FCC) Structure

The face-centered cubic (FCC) structure is a type of crystal lattice where atoms are located at each of the corners and the centers of all the faces of the cube. In an FCC unit cell, there are a total of four atoms per unit cell, contributing to its high packing efficiency. Understanding this structure is essential for calculating properties like edge length and density.

Unit Cell Edge Length

The edge length of a unit cell in a crystal lattice is the length of one side of the cube that defines the repeating unit of the crystal structure. For FCC lattices, the relationship between the atomic radius and the edge length can be expressed as a = 2√2r, where 'a' is the edge length and 'r' is the atomic radius. This relationship is crucial for determining the dimensions of the unit cell.

Density Calculation

Density is defined as mass per unit volume and is calculated using the formula density = mass/volume. For a crystalline solid, the mass can be determined from the number of atoms in the unit cell and the atomic mass, while the volume is derived from the cube of the edge length. This concept is vital for finding the density of platinum once the edge length is known.