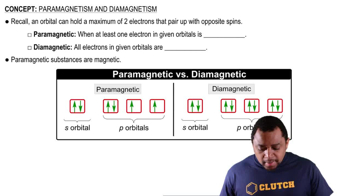
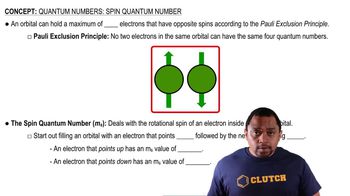
Nickel(II) complexes with the formula NiX2L2, where X− is Cl− or N-bonded NCS− and L is the monodentate triphenylphosphine ligand P(C6H5)3, can be square planar or tetrahedral.
(a) Draw crystal field energy-level diagrams for a square planar and a tetrahedral nickel(II) complex, and show the population of the orbitals.