Here are the essential concepts you must grasp in order to answer the question correctly.
Binding Energy
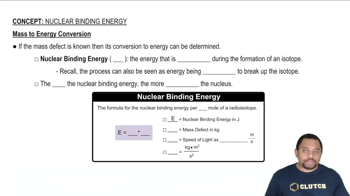
Binding energy is the energy required to disassemble a nucleus into its constituent protons and neutrons. It reflects the stability of the nucleus; a higher binding energy indicates a more stable nucleus. This energy can be calculated using the mass defect, which is the difference between the mass of the individual nucleons and the actual mass of the nucleus.
Recommended video:
Mass Defect
The mass defect is the difference between the total mass of the separate nucleons (protons and neutrons) and the mass of the nucleus itself. This mass loss, due to the conversion of mass into energy according to Einstein's equation E=mc², is crucial for calculating the binding energy. It highlights how energy is released when nucleons bind together to form a nucleus.
Recommended video:
Energy Conversion
In nuclear physics, energy is often expressed in different units, such as joules and MeV (mega-electronvolts). To convert the binding energy from joules to MeV, one must use the conversion factor where 1 MeV equals 1.60 x 10^-13 joules. Understanding this conversion is essential for accurately reporting binding energy in the context of nuclear reactions and stability.
Recommended video: