Here are the essential concepts you must grasp in order to answer the question correctly.
Nuclear Fusion
Nuclear fusion is a process where two light atomic nuclei combine to form a heavier nucleus, releasing energy in the process. This reaction is the source of energy for stars, including the sun, and occurs under extreme temperature and pressure conditions. In the context of the question, the fusion of deuterium (2H) to form helium-3 (3He) is a specific example of this phenomenon.
Recommended video:
Mass-Energy Equivalence
Mass-energy equivalence, expressed by Einstein's equation E=mc², states that mass can be converted into energy and vice versa. In nuclear reactions, the mass of the products is often less than the mass of the reactants, and this 'missing' mass is converted into energy. Understanding this concept is crucial for calculating the energy released in the fusion reaction described in the question.
Recommended video:
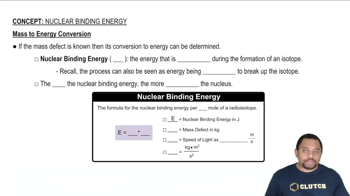
Energy to Mass Conversion
Atomic Mass and Energy Calculation
Atomic mass is the mass of an atom, typically measured in atomic mass units (amu), and is essential for calculating the energy released in nuclear reactions. To find the energy released during fusion, one must determine the mass defect (the difference in mass between reactants and products) and convert this mass defect into energy using the mass-energy equivalence principle. This calculation is key to answering the question accurately.
Recommended video:
 Verified step by step guidance
Verified step by step guidance