One of the emission lines of the hydrogen atom has a wavelength of 93.07 nm. b. Determine the initial and final values of n associated with this emission.

Verified step by step guidance

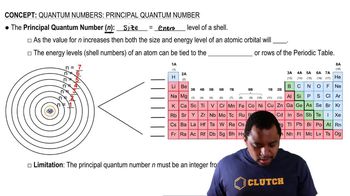
1. insert step 1> Identify that the problem involves the hydrogen atom emission spectrum, which can be analyzed using the Rydberg formula for hydrogen: \( \frac{1}{\lambda} = R_H \left( \frac{1}{n_1^2} - \frac{1}{n_2^2} \right) \), where \( \lambda \) is the wavelength, \( R_H \) is the Rydberg constant (1.097 \times 10^7 \text{ m}^{-1}), and \( n_1 \) and \( n_2 \) are the principal quantum numbers with \( n_2 > n_1 \).
2. insert step 2> Convert the given wavelength from nanometers to meters for consistency with the Rydberg constant: \( 93.07 \text{ nm} = 93.07 \times 10^{-9} \text{ m} \).
3. insert step 3> Rearrange the Rydberg formula to solve for \( \frac{1}{n_1^2} - \frac{1}{n_2^2} \): \( \frac{1}{n_1^2} - \frac{1}{n_2^2} = \frac{1}{\lambda R_H} \). Substitute the values of \( \lambda \) and \( R_H \) into the equation.
4. insert step 4> Calculate the right-hand side of the equation to find the numerical value of \( \frac{1}{n_1^2} - \frac{1}{n_2^2} \).
5. insert step 5> Use trial and error or logical deduction to find integer values of \( n_1 \) and \( n_2 \) that satisfy the equation, keeping in mind that \( n_2 > n_1 \). Common transitions in the hydrogen spectrum involve \( n_1 = 1, 2, 3, \ldots \) and \( n_2 = 2, 3, 4, \ldots \).

Key Concepts

Emission Spectra

Emission spectra are produced when electrons in an atom transition from a higher energy level to a lower one, releasing energy in the form of light. Each element has a unique emission spectrum, which can be used to identify the element. In the case of hydrogen, the emission lines correspond to specific wavelengths that relate to the energy differences between quantized electron orbits.

Quantum Numbers

Quantum numbers are values that describe the energy levels and positions of electrons in an atom. The principal quantum number (n) indicates the main energy level of an electron, with higher values of n corresponding to higher energy levels. For hydrogen, transitions between these levels result in the emission of photons with specific wavelengths, which can be calculated using the Rydberg formula.

Rydberg Formula

The Rydberg formula is used to predict the wavelengths of spectral lines in hydrogen and other hydrogen-like atoms. It is expressed as 1/λ = R_H (1/n_f^2 - 1/n_i^2), where λ is the wavelength, R_H is the Rydberg constant, n_f is the final energy level, and n_i is the initial energy level. By rearranging this formula, one can determine the quantum numbers associated with a given wavelength.