The decomposition reaction of N2O5 in carbon tetrachloride is 2 N2O5¡4 NO2 + O2. The rate law is first order in N2O5. At 64 C the rate constant is 4.82 * 10-3 s-1. (a) Write the rate law for the reaction.
The react ion between ethyl bromide 1C2H5Br2 and hydroxide ion in ethyl alcohol at 330 K, C2H5Br1alc2 + OH- 1alc2¡ C2H5OH1l2 + Br - 1alc2, is first order each in ethyl bromide and hydroxide ion. When 3C2H5Br4 is 0.0477 M and 3OH- 4 is 0.100 M, the rate of disappearance of ethyl bromide is 1.7 * 10-7 M>s. (c) How would the rate of disappearance of ethyl bromide change if the solution were diluted by adding an equal volume of pure ethyl alcohol to the solution?
 Verified step by step guidance
Verified step by step guidance
Verified Solution
Key Concepts
Rate Law

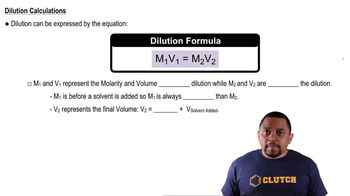
Dilution Effect
First-Order Reactions

Consider the following reaction: 2 NO1g2 + 2 H21g2¡N21g2 + 2 H2O1g2 (d) What is the reaction rate at 1000 K if [NO] is decreased to 0.010 M and 3H24 is increased to 0.030 M?
The react ion between ethyl bromide 1C2H5Br2 and hydroxide ion in ethyl alcohol at 330 K, C2H5Br1alc2 + OH- 1alc2¡ C2H5OH1l2 + Br - 1alc2, is first order each in ethyl bromide and hydroxide ion. When 3C2H5Br4 is 0.0477 M and 3OH- 4 is 0.100 M, the rate of disappearance of ethyl bromide is 1.7 * 10-7 M>s. (a) What is the value of the rate constant?
The iodide ion reacts with hypochlorite ion (the active ingredient in chlorine bleaches) in the following way: OCl- + I- → OI- + Cl- . This rapid reaction gives the following rate data:
[OCl4-] (M) [I-] (M) Initial Rate (M,s)
1.5 * 10-3 1.5 * 10-3
1.36 * 10-4 3.0 * 10-3 1.5 * 10-3 2.72 * 10-4
1.5 * 10-3 3.0 * 10-3 2.72 * 10-4
(a) Write the rate law for this reaction.
The iodide ion reacts with hypochlorite ion (the active ingredient in chlorine bleaches) in the following way: OCl - + I - ¡OI - + Cl - . This rapid reaction gives the following rate data:
[OCl4-] (M) [I-] (M) Initial Rate (M,s)
1.5 * 10-3 1.5 * 10-3
1.36 * 10-4 3.0 * 10-3 1.5 * 10-3 2.72 * 10-4
1.5 * 10-3 3.0 * 10-3 2.72 * 10-4
(b) Calculate the rate constant with proper units.
The iodide ion reacts with hypochlorite ion (the active ingredient in chlorine bleaches) in the following way: OCl- + I- → OI- + Cl- . This rapid reaction gives the following rate data:
[OCl4-] (M) [I-] (M) Initial Rate (M,s)
1.5 * 10-3 1.5 * 10-3
1.36 * 10-4 3.0 * 10-3 1.5 * 10-3 2.72 * 10-4
1.5 * 10-3 3.0 * 10-3 2.72 * 10-4 (c) Calculate the rate when [OCl-] = 2.0 * 10-3 M and [I-] = 5.0 * 10 - 4 M.
