Which of the three-dimensional primitive lattices has a unit cell where none of the internal angles is 90? (a) Orthorhombic, (b) hexagonal, (c) rhombohedral, (d) triclinic, (e) both rhombohedral and triclinic.
The unit cell of a compound containing potassium, aluminum, and fluorine is shown here. (a) What type of lattice does this crystal possess (all three lattice vectors are mutually perpendicular)?

 Verified step by step guidance
Verified step by step guidance
Verified Solution
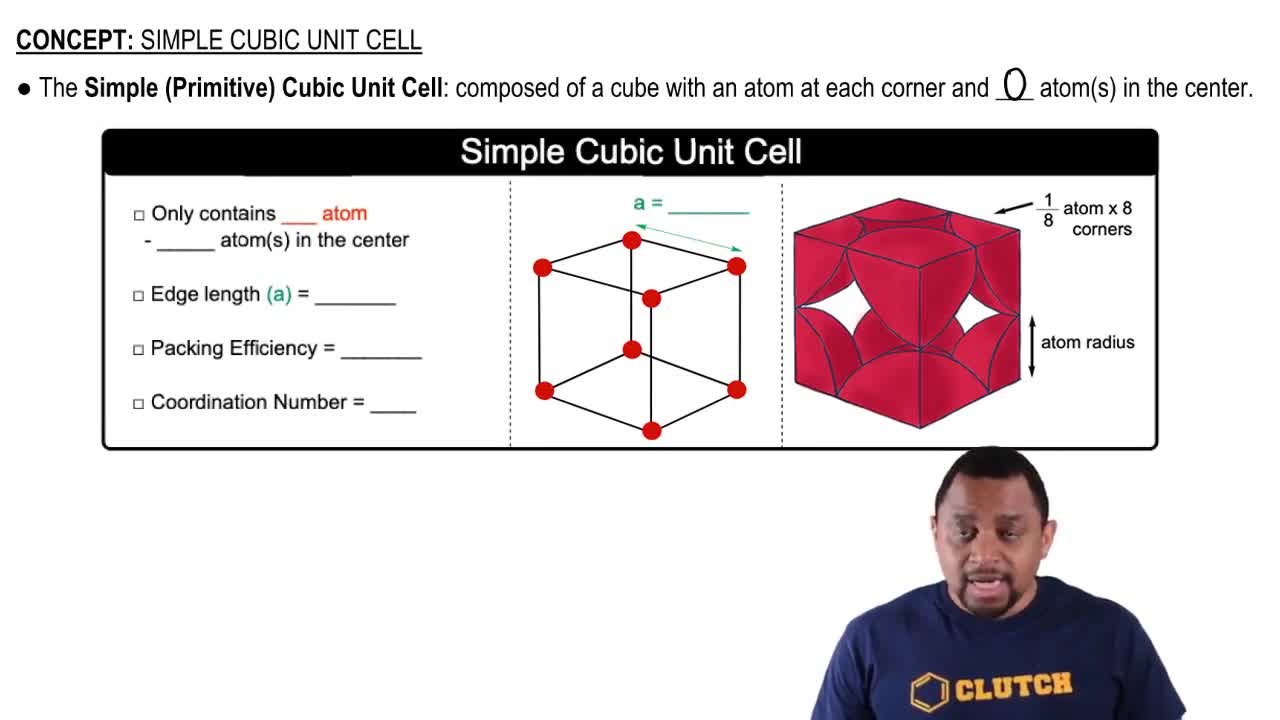
Key Concepts
Crystal Lattice

Unit Cell Parameters
Types of Crystal Lattices

What is the minimum number of atoms that could be contained in the unit cell of an element with a face-centered cubic lattice? (a) 1, (b) 2, (c) 3, (d) 4, (e) 5.
The unit cell of nickel arsenide is shown here. (b) What is the empirical formula?
Consider the unit cells shown here for three different structures that are commonly observed for metallic elements. (a) Which structure(s) corresponds to the densest packing of atoms?
Consider the unit cells shown here for three different structures that are commonly observed for metallic elements. (b) Which structure(s) corresponds to the least dense packing of atoms?
Sodium metal (atomic weight 22.99 g>mol) adopts a body-centered cubic structure with a density of 0.97 g>cm3. (b) If sodium didn't react so vigorously, it could float on water. Use the answer from part (a) to estimate the density of Na if its structure were that of a cubic close-packed metal. Would it still float on water?
