The Lyman series of emission lines of the hydrogen atom are those for which nf = 1. (b) Calculate the wavelengths of the first three lines in the Lyman series—those for which ni = 2, 3, and 4.
Ch.6 - Electronic Structure of Atoms
Chapter 6, Problem 44b
The hydrogen atom can absorb light of wavelength 1094 nm. (b) Determine the final value of n associated with this absorption.

Verified Solution
Video duration:
2mWas this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Energy of Photons
The energy of a photon is directly related to its wavelength, described by the equation E = hc/λ, where E is energy, h is Planck's constant, c is the speed of light, and λ is the wavelength. For the hydrogen atom, when it absorbs a photon, the energy from the photon is used to excite an electron to a higher energy level.
Recommended video:
Guided course

Photon Energy Formulas
Quantum Energy Levels
In a hydrogen atom, electrons occupy discrete energy levels, denoted by the principal quantum number n. The energy associated with each level can be calculated using the formula E_n = -13.6 eV/n². When a photon is absorbed, the electron transitions from a lower energy level (n_initial) to a higher one (n_final), and the difference in energy corresponds to the energy of the absorbed photon.
Recommended video:
Guided course
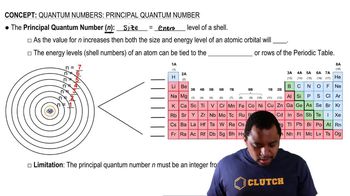
Principal Quantum Number
Balmer and Rydberg Formulas
The Rydberg formula allows for the calculation of the wavelengths of spectral lines in hydrogen and is given by 1/λ = R_H(1/n_final² - 1/n_initial²). This formula is essential for determining the final energy level (n_final) after absorption, as it relates the wavelength of light absorbed to the transition between quantum states of the hydrogen atom.
Recommended video:
Guided course

Balmer Series Example
Related Practice
Textbook Question
2645
views
Textbook Question
One of the emission lines of the hydrogen atom has a wavelength of 94.974 nm. (a) In what region of the electromagnetic spectrum is this emission found?
508
views
Textbook Question
One of the emission lines of the hydrogen atom has a wavelength of 94.974 nm. (b) Determine the initial and final values of n associated with this emission.
1895
views
1
comments
Textbook Question
Order the following transitions in the hydrogen atom from smallest to largest frequency of light absorbed: n = 3 to n = 7, n = 4 to n = 8, n = 2 to n = 5, and n = 1 to n = 3.
3444
views
Textbook Question
Write the electron configurations for the following ions, and
determine which have noble-gas configurations:
(a) Ti2+
(b) Br-
(c) Mg2+
(d) Po2-
(e) Pt2+
(f) V3+
1433
views
Textbook Question
Use the de Broglie relationship to determine the wavelengths of the following objects: (a) an 85-kg person skiing at 50 km/hr
695
views
