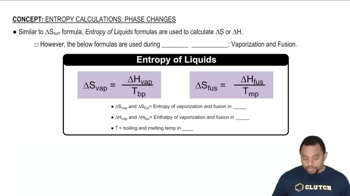
Textbook Question
(a) Does the entropy of the surroundings increase for spontaneous processes?
567
views



(a) Does the entropy of the surroundings increase for spontaneous processes?
(b) In a particular spontaneous process the entropy of the system decreases. What can you conclude about the sign and magnitude of ΔSsurr?
(c) During a certain reversible process, the surroundings undergo an entropy change, ΔSsurr = -78 J/K. What is the entropy change of the system for this process?
For the isothermal expansion of a gas into a vacuum, ΔE = 0, q = 0, and w = 0. (b) Explain why no work is done by the system during this process.
(a) What is the difference between a state and a microstate of a system?
(b) As a system goes from state A to state B, its entropy decreases. What can you say about the number of microstates corresponding to each state?