Here are the essential concepts you must grasp in order to answer the question correctly.
Factoring Quadratic Equations
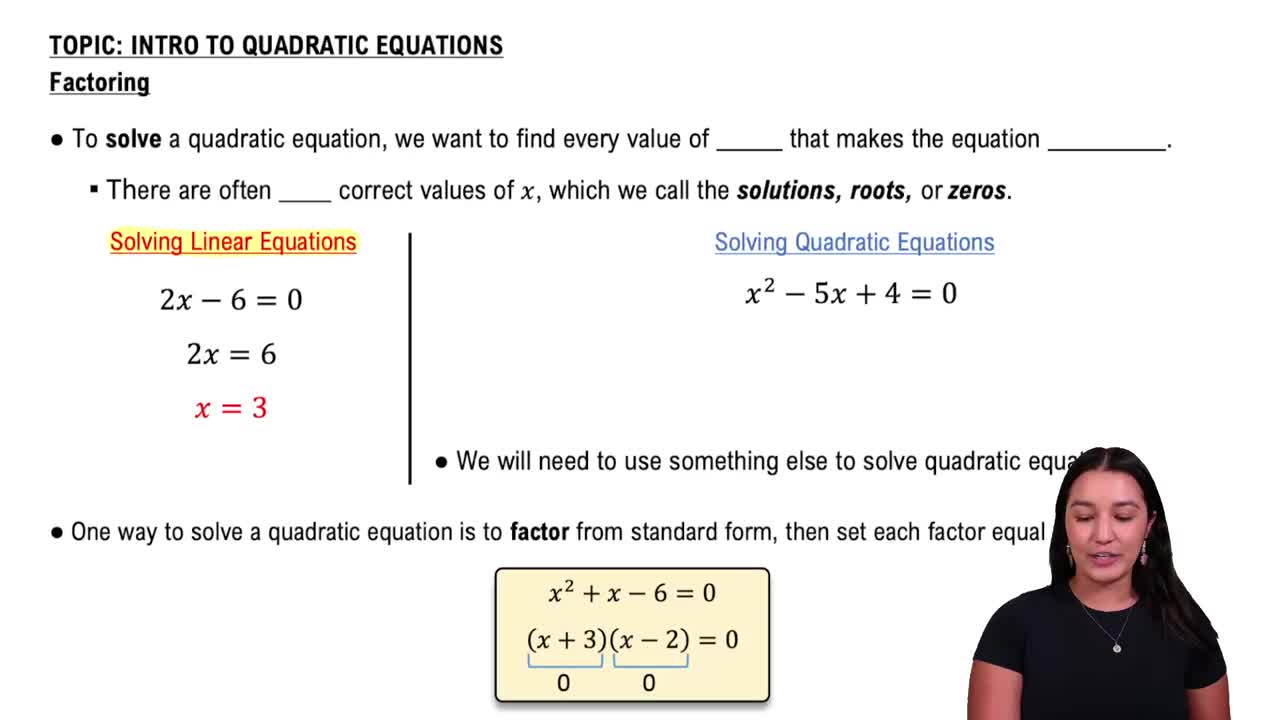
Factoring is a method used to solve quadratic equations by expressing them as a product of their linear factors. For a quadratic equation in the form ax^2 + bx + c = 0, we look for two numbers that multiply to ac and add to b. This allows us to rewrite the equation in a factorable form, making it easier to find the roots.
Recommended video:
Solving Quadratic Equations by Factoring
Zero Product Property
The Zero Product Property states that if the product of two factors equals zero, then at least one of the factors must be zero. This principle is crucial when solving factored equations, as it allows us to set each factor equal to zero and solve for the variable, leading to the solutions of the original equation.
Recommended video:
Product, Quotient, and Power Rules of Logs
Quadratic Formula
The Quadratic Formula, x = (-b ± √(b² - 4ac)) / (2a), provides a method for finding the roots of any quadratic equation. While the question specifically asks for factoring, understanding this formula is essential as it serves as a backup method for solving quadratics that may not factor easily, ensuring that all possible solutions can be found.
Recommended video:
Solving Quadratic Equations Using The Quadratic Formula