In Exercises 1–26, solve and check each linear equation. 2 - (7x + 5) = 13 - 3x

Verified step by step guidance

1. Distribute the negative sign on the left side: 2 - 7x - 5 = 13 - 3x.
2. Combine like terms on the left side: -7x - 3 = 13 - 3x.
3. Add 7x to both sides to get all x terms on one side: -3 = 13 + 4x.
4. Subtract 13 from both sides to isolate the x term: -16 = 4x.
5. Divide both sides by 4 to solve for x: x = -4.

Key Concepts

Linear Equations

A linear equation is an algebraic expression that represents a straight line when graphed. It typically takes the form ax + b = c, where a, b, and c are constants, and x is the variable. Solving a linear equation involves isolating the variable on one side of the equation to find its value.

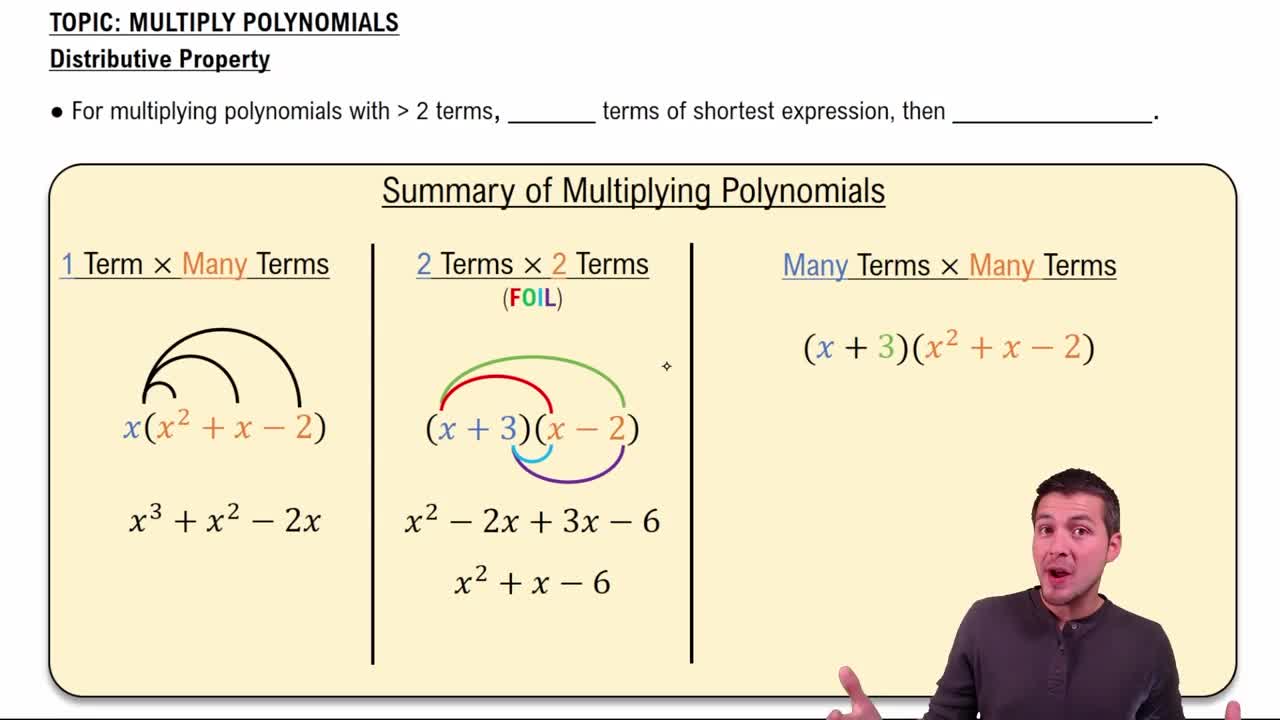
Distributive Property

The distributive property states that a(b + c) = ab + ac, allowing us to multiply a single term by two or more terms inside parentheses. This property is essential for simplifying expressions and solving equations, as it helps eliminate parentheses and combine like terms effectively.

Checking Solutions

Checking solutions involves substituting the found value of the variable back into the original equation to verify its correctness. This step ensures that the solution satisfies the equation, confirming that no errors occurred during the solving process. It is a crucial part of problem-solving in algebra.