Textbook Question
Solve each triangle. Approximate values to the nearest tenth.
<IMAGE>
213
views
 Verified step by step guidance
Verified step by step guidance

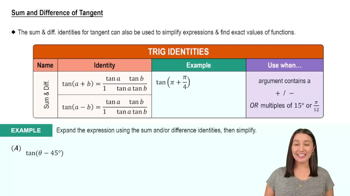

Solve each triangle. Approximate values to the nearest tenth.
<IMAGE>
Vector v has the given direction angle and magnitude. Find the horizontal and vertical components.
θ = 50°, |v| = 26
Solve each triangle ABC.
<IMAGE>
Find the unknown angles in triangle ABC for each triangle that exists.
B = 74.3°, a = 859 m, b = 783 m
Vector v has the given direction angle and magnitude. Find the horizontal and vertical components.
θ = 27° 30' |v| = 15.4
Solve each triangle. Approximate values to the nearest tenth.
<IMAGE>