Here are the essential concepts you must grasp in order to answer the question correctly.
Complex Numbers
Complex numbers are numbers that have a real part and an imaginary part, expressed in the form a + bi, where a is the real part, b is the imaginary part, and i is the imaginary unit defined as the square root of -1. Understanding complex numbers is essential for performing operations involving them, such as addition, subtraction, multiplication, and division.
Recommended video:
Squaring a Binomial
Squaring a binomial involves applying the formula (a - b)² = a² - 2ab + b². This formula is crucial for expanding expressions like (3 - 4i)², as it allows us to systematically calculate the square of the binomial by squaring each term and accounting for the product of the two terms.
Recommended video:
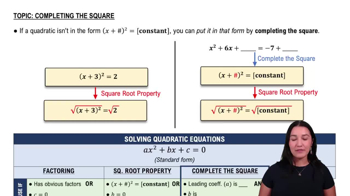
Solving Quadratic Equations by Completing the Square
Standard Form of Complex Numbers
The standard form of a complex number is expressed as a + bi, where a and b are real numbers. When performing operations on complex numbers, it is important to express the final result in this form to clearly identify the real and imaginary components, facilitating further calculations and interpretations.
Recommended video:
Complex Numbers In Polar Form
 Blitzer 3rd Edition
Blitzer 3rd Edition Ch. 5 - Complex Numbers, Polar Coordinates and Parametric Equations
Ch. 5 - Complex Numbers, Polar Coordinates and Parametric Equations Problem 4
Problem 4 Verified step by step guidance
Verified step by step guidance