Here are the essential concepts you must grasp in order to answer the question correctly.
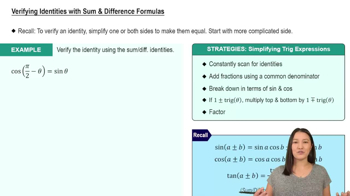
Sum and Difference Identities
The sum and difference identities are formulas that express the sine, cosine, and tangent of the sum or difference of two angles in terms of the sine and cosine of the individual angles. For example, the sine difference identity states that sin(A - B) = sin(A)cos(B) - cos(A)sin(B). These identities are essential for simplifying trigonometric expressions and finding exact values.
Recommended video:
Verifying Identities with Sum and Difference Formulas
Exact Values of Trigonometric Functions
Exact values of trigonometric functions refer to the specific values of sine, cosine, and tangent for commonly used angles, such as 0°, 30°, 45°, 60°, and 90°. Knowing these values allows for quick calculations and simplifications in trigonometric problems. For instance, sin(60°) = √3/2 and cos(45°) = √2/2 are examples of exact values that can be used in conjunction with identities.
Recommended video:
Introduction to Trigonometric Functions
Angle Measurement in Degrees
Angle measurement in degrees is a way to quantify angles, where a full circle is divided into 360 equal parts. In trigonometry, angles are often expressed in degrees, and understanding how to convert between degrees and radians is crucial. For example, 60° and 45° are both angles that can be directly used in trigonometric calculations, particularly when applying sum and difference identities.
Recommended video:
Reference Angles on the Unit Circle
 Verified step by step guidance
Verified step by step guidance