Here are the essential concepts you must grasp in order to answer the question correctly.
Product-to-Sum Formulas
Product-to-sum formulas are trigonometric identities that allow the transformation of products of sine and cosine functions into sums or differences. For example, the formula for sin(x)cos(y) can be expressed as (1/2)[sin(x+y) + sin(x-y)]. These formulas simplify calculations and are essential for integrating or differentiating trigonometric expressions.
Recommended video:
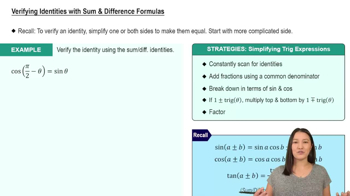
Verifying Identities with Sum and Difference Formulas
Trigonometric Identities
Trigonometric identities are equations that hold true for all values of the variables involved, particularly angles. They include fundamental identities like the Pythagorean identity, reciprocal identities, and co-function identities. Understanding these identities is crucial for manipulating and simplifying trigonometric expressions in various problems.
Recommended video:
Fundamental Trigonometric Identities
Angle Addition and Subtraction
Angle addition and subtraction formulas express the sine and cosine of the sum or difference of two angles in terms of the sines and cosines of the individual angles. For instance, sin(a ± b) = sin(a)cos(b) ± cos(a)sin(b). These formulas are vital for solving problems involving the combination of angles, particularly in the context of the product-to-sum transformation.
Recommended video:
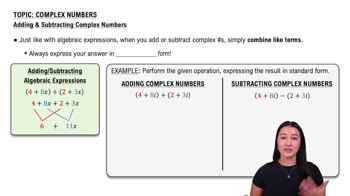
Adding and Subtracting Complex Numbers
 Verified step by step guidance
Verified step by step guidance