Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
3. Unit Circle
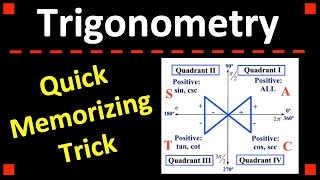
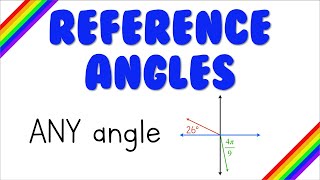
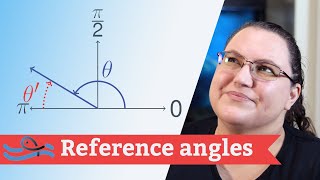
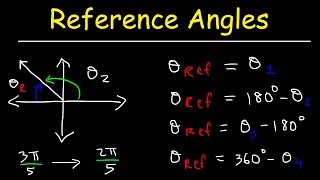
Reference Angles
Problem 83
Textbook Question
Textbook QuestionIn Exercises 61–86, use reference angles to find the exact value of each expression. Do not use a calculator. tan (-17𝜋/6)
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
5mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
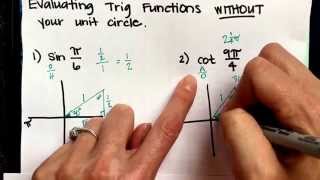
Reference Angles
A reference angle is the acute angle formed by the terminal side of an angle in standard position and the x-axis. It is always positive and is used to simplify the calculation of trigonometric functions for angles that are not in the first quadrant. For angles greater than 360 degrees or less than 0 degrees, the reference angle can be found by determining the equivalent angle within the range of 0 to 2π.
Recommended video:

Reference Angles on the Unit Circle
Tangent Function
The tangent function, denoted as tan(θ), is defined as the ratio of the opposite side to the adjacent side in a right triangle. It can also be expressed in terms of sine and cosine as tan(θ) = sin(θ)/cos(θ). Understanding the properties of the tangent function, including its periodicity and behavior in different quadrants, is essential for evaluating tangent values for various angles.
Recommended video:

Introduction to Tangent Graph
Angle Coterminality
Coterminal angles are angles that share the same terminal side when drawn in standard position. To find a coterminal angle, you can add or subtract multiples of 2π (or 360 degrees). For example, to find a coterminal angle for -17π/6, you would add 2π until the angle is within the standard range of 0 to 2π, which helps in simplifying the evaluation of trigonometric functions.
Recommended video:
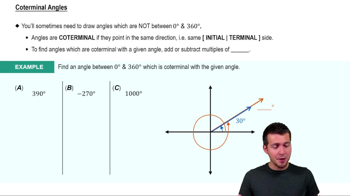
Coterminal Angles

 5:31m
5:31mWatch next
Master Reference Angles on the Unit Circle with a bite sized video explanation from Callie Rethman
Start learningRelated Videos
Related Practice