Determine whether each statement is possible or impossible. b. tan θ = 1.4

Verified step by step guidance

1. <insert step 1> Identify the range of the tangent function. The tangent function, \( \tan \theta \), can take any real number value from \(-\infty\) to \(+\infty\).>
2. <insert step 2> Recognize that \( \tan \theta = 1.4 \) is a real number.>
3. <insert step 3> Since 1.4 is within the range of possible values for the tangent function, the statement \( \tan \theta = 1.4 \) is possible.>
4. <insert step 4> Consider the unit circle or the tangent graph to visualize that \( \tan \theta \) can indeed equal 1.4 at some angle \( \theta \).>
5. <insert step 5> Conclude that the statement is possible because the tangent function can take any real number value, including 1.4.>

Key Concepts

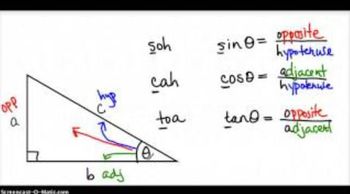
Tangent Function

The tangent function, denoted as tan(θ), is a fundamental trigonometric function defined as the ratio of the opposite side to the adjacent side in a right triangle. It can also be expressed as tan(θ) = sin(θ) / cos(θ). The range of the tangent function is all real numbers, meaning it can take any value, including positive values like 1.4.

Angle Measurement

Angles in trigonometry can be measured in degrees or radians. The tangent function is periodic with a period of π radians (or 180 degrees), which means that for any angle θ, tan(θ) = tan(θ + nπ) for any integer n. This periodicity allows for multiple angles to yield the same tangent value, including 1.4.

Existence of Solutions

In trigonometry, a statement like tan(θ) = 1.4 is possible because the tangent function can produce any real number as an output. Therefore, there exist angles θ for which this equation holds true. Specifically, one can find angles in both the first and third quadrants that satisfy this condition.