Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
6. Trigonometric Identities and More Equations
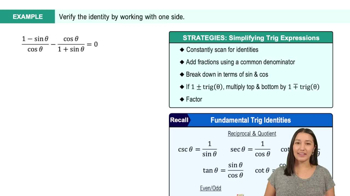
Introduction to Trigonometric Identities
Problem 49
Textbook Question
Textbook QuestionIn Exercises 47–54, use the figures to find the exact value of each trigonometric function. θ tan ------- 2
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Trigonometric Functions
Trigonometric functions relate the angles of a triangle to the lengths of its sides. The primary functions include sine (sin), cosine (cos), and tangent (tan), which are defined as ratios of the sides of a right triangle. Understanding these functions is essential for solving problems involving angles and distances in trigonometry.
Recommended video:

Introduction to Trigonometric Functions
Tangent Function
The tangent function is defined as the ratio of the opposite side to the adjacent side in a right triangle. It can also be expressed in terms of sine and cosine as tan(θ) = sin(θ) / cos(θ). Knowing how to calculate and interpret the tangent function is crucial for finding exact values in trigonometric problems.
Recommended video:

Introduction to Tangent Graph
Exact Values of Trigonometric Functions
Exact values of trigonometric functions refer to specific values that can be determined without approximation, often using special angles like 0°, 30°, 45°, 60°, and 90°. These values are typically derived from the unit circle or special right triangles, and they are fundamental for solving trigonometric equations and problems accurately.
Recommended video:

Introduction to Trigonometric Functions

 6:19m
6:19mWatch next
Master Even and Odd Identities with a bite sized video explanation from Callie Rethman
Start learningRelated Videos
Related Practice