Table of contents
- 0. Review of College Algebra4h 43m
- 1. Measuring Angles39m
- 2. Trigonometric Functions on Right Triangles2h 5m
- 3. Unit Circle1h 19m
- 4. Graphing Trigonometric Functions1h 19m
- 5. Inverse Trigonometric Functions and Basic Trigonometric Equations1h 41m
- 6. Trigonometric Identities and More Equations2h 34m
- 7. Non-Right Triangles1h 38m
- 8. Vectors2h 25m
- 9. Polar Equations2h 5m
- 10. Parametric Equations1h 6m
- 11. Graphing Complex Numbers1h 7m
4. Graphing Trigonometric Functions
Graphs of the Sine and Cosine Functions
Problem 51a
Textbook Question
Textbook QuestionDetermine whether each function is even, odd, or neither. See Example 5. ƒ(x) = 0.5x⁴ - 2x² + 6
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Even and Odd Functions
Even functions are symmetric about the y-axis, meaning that f(x) = f(-x) for all x in the domain. Odd functions have rotational symmetry about the origin, satisfying the condition f(-x) = -f(x). A function can also be neither even nor odd if it does not meet either condition.
Recommended video:

Even and Odd Identities
Polynomial Functions
Polynomial functions are expressions that consist of variables raised to non-negative integer powers and multiplied by coefficients. The degree of the polynomial, determined by the highest power of x, influences its behavior and symmetry. In this case, the function is a polynomial of degree 4.
Recommended video:
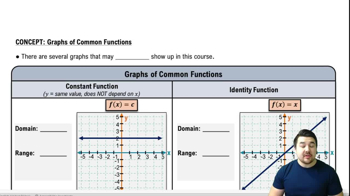
Graphs of Common Functions
Testing for Symmetry
To determine if a function is even or odd, substitute -x into the function and simplify. If the result equals the original function, it is even; if it equals the negative of the original function, it is odd. If neither condition holds, the function is classified as neither even nor odd.
Recommended video:

Cardioids

 5:53m
5:53mWatch next
Master Graph of Sine and Cosine Function with a bite sized video explanation from Nick Kaneko
Start learningRelated Videos
Related Practice