The Number e - Online Tutor, Practice Problems & Exam Prep

Exponential functions with base e (approximately 2.71828) can be evaluated and graphed similarly to other bases. For example, to evaluate f(x) = e^x, use a calculator with the second ln function. The significance of e arises from its role in compounding interest and applications in population growth and radioactive decay. Understanding e enhances comprehension of various mathematical and real-world phenomena, making it a crucial constant in exponential functions.

The Number e

Hey, everyone. When working through problems with different exponential expressions and exponential functions, you may come across one that doesn't have a base of something like 2 or 1 half or ten or any other number but actually has a base of e, this lowercase e. And the first time you see that, you might be wondering why there is another letter in that function when we already have x right there and how we are going to work with this function with 2 different variables. But you don't have to worry about any of that because here I'm going to show you how e is literally just a number, and we can treat it just like we would any other exponential function and evaluate and graph it using all of the tools that we already know. So let's go ahead and get started. Now, like I said, e is not a variable at all but simply a number. And another similar number that you might be a bit more familiar with is pi. We know that pi is 3.1415 and so on, this long decimal that we don't write out. We just write pi. E is really similar. It's this long decimal, 2.71828 and so on, but we simply write it as e. Now because it's a number, we can treat it just like we would any other exponential function and do things like evaluate it for different values of x. So let's take a look at our function here, \( f(x) = e^x \), and go ahead and evaluate this for \( x = 2 \). Now I'm simply going to plug 2 in for x into my function, so \( f(2) = e^2 \). Now when working with exponential functions with base \( e \), we do want to use a calculator to evaluate these. And the buttons that you're going to use on your calculator in order to get this base \( e \) are second ln. This should give you \( e \) raised to the power of, and then you simply type in what your power is. So for \( e^2 \), I would type second ln and then 2 in order to get my answer, rounding to the nearest 100th place, which would be 7.39. Now this would be my final answer here, but let's go ahead and evaluate this function for \( x = -3 \). Now, again, we're just going to be plugging in negative 3 for x here. So \( f(-3) = e^{-3} \), which knowing our rules for exponents, this would really just be \( \frac{1}{e^3} \). And you can type either of these into your calculator, and you should get the same answer. So typing \( e^{-3} \), I would type second ln and then negative 3. And rounding to the nearest hundredths place, I would get an answer of 0.05 as my final answer here. Now we can evaluate exponential functions of base e like any other exponential function, and we can also graph them just like we would any other exponential function as well. So if I take my function here, \( e^x \), I know that my graph is going to have the exact same shape as any other exponential function. So my graph is going to end up looking something like this, \( f(x) = e^x \). And you see here that it's right in between my graphs of \( 2^x \) and \( 3^x \), which this happens because e, we know, is this number, 2.718 and so on, which happens to be right in between 2 and 3. So I know that my number e is right in between 2 and 3, so it makes sense that the graph of my function \( e^x \) is right in between the graphs of the exponential functions with base 2 and base 3. Now if you're faced with graphing a more complicated function of base e, you can simply graph it using transformations, the same method that we used for any other more complicated functions of different bases like 2 or 3. Now hopefully, with all of this, you see that we can treat exponential functions of base e just like any other exponential function no matter what the scenario is. But you still might be wondering why we need this base of e in the first place. Why do we need to have this base e when we have all these other numbers to choose from that aren't crazy decimals? So I'm going to give you a little bit more information about what e is and where it comes from. So e actually comes from the idea of compounding interest, which is this equation right here. And we want our interest to compound as much as possible. So if I take the number of times that my interest is compounded, this n, and take this all the way up to infinity, this equation is going to end up giving me 2.71828 and so on, which we know is just e. Now, that's where e comes from, compounding interest, but it's actually going to pop up in a ton of other stuff that you'll see. Now, you might see in your other courses that e is a part of predicting population growth and working with something like radioactive decay and half-lives. So e is just a number, but it's a number that describes a bunch of different things going on in the world. And even with describing all of these different things and being super useful, we can treat it just like we would any other exponential function. So with that in mind, hopefully, you have a better idea of what e is and why we need it and how exactly to work with it. Thanks for watching, and let me know if you have any questions.

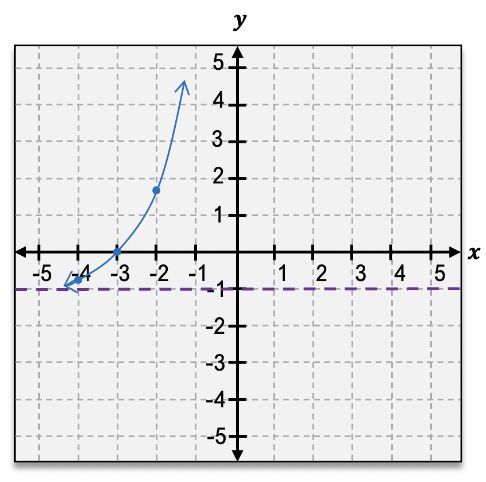
Graph the given function.

$g\left(x\right)=e^{x+3}-1$

• A.
• B.
• C.
• D.

Here’s what students ask on this topic:

What is the number e and why is it important in mathematics?

The number e, approximately 2.71828, is a mathematical constant that is the base of natural logarithms. It is important because it arises naturally in various contexts, such as in the calculation of compound interest, population growth models, and radioactive decay. The number e is unique in that the function e^x has the same rate of growth as its value, making it crucial in calculus and differential equations. Understanding e helps in comprehending exponential growth and decay processes in both mathematics and real-world applications.

How do you evaluate an exponential function with base e using a calculator?

To evaluate an exponential function with base e, such as f(x) = e^x, using a calculator, follow these steps: First, locate the 'e' function on your calculator, which is often accessed by pressing the 'second' or 'shift' key followed by the 'ln' key. Then, enter the exponent value. For example, to evaluate e², press 'second', 'ln', and then '2'. The calculator will display the result, which is approximately 7.39 when rounded to the nearest hundredth. This method allows you to compute values of e raised to any power efficiently.

How do you graph an exponential function with base e?

To graph an exponential function with base e, such as f(x) = e^x, follow these steps: First, create a table of values by choosing several x-values and calculating the corresponding y-values using the function. Plot these points on a coordinate plane. The graph of e^x will have a characteristic shape, starting close to the x-axis for negative x-values and increasing rapidly for positive x-values. The graph will pass through the point (0,1) since e⁰ = 1. The curve will be asymptotic to the x-axis as x approaches negative infinity and will increase without bound as x approaches positive infinity.

What are some real-world applications of the number e?

The number e has numerous real-world applications. It is used in calculating compound interest, where interest is compounded continuously. In biology, e is used in models of population growth, where populations grow exponentially. In physics, e appears in the equations describing radioactive decay and half-lives. Additionally, e is used in various fields of engineering, economics, and statistics, such as in the analysis of growth rates and in the calculation of probabilities in certain distributions. Its natural occurrence in these diverse areas highlights its significance in both theoretical and applied mathematics.

Why is the number e used as the base for natural logarithms?

The number e is used as the base for natural logarithms because it simplifies many mathematical expressions and calculations. The natural logarithm, denoted as ln(x), is the inverse function of the exponential function e^x. Using e as the base ensures that the derivative of e^x is simply e^x, and the derivative of ln(x) is 1/x. This property makes calculus operations involving exponential and logarithmic functions more straightforward. Additionally, e naturally arises in various growth and decay processes, making it a convenient and practical choice for the base of natural logarithms.