Asymptotes - Online Tutor, Practice Problems & Exam Prep

Understanding rational functions involves identifying asymptotes, which are lines the graph approaches but never touches. Vertical asymptotes occur where the denominator equals zero, while horizontal asymptotes depend on the degrees of the numerator and denominator. For instance, if the degree of the numerator is less than that of the denominator, the horizontal asymptote is at $0$. Additionally, common factors can create holes in the graph, indicating removable discontinuities. Mastering these concepts is essential for graphing rational functions accurately.

Introduction to Asymptotes

Hey everyone. Whenever we worked with polynomial functions and we graphed them, we had to consider the end behavior of our graph, and this gave us great information so that we could easily graph our polynomial function. Now with rational functions, we're going to have to consider the behavior of our graph around things called asymptotes. Now asymptotes are lines that our curve or our graph is going to approach and get really, really close to but not quite touch. Just that word, asymptote, might make the graphs of rational functions sound like they're going to be complicated, but here we're going to graph a rational function together and I'm going to walk you through identifying asymptotes and the basics that you need to know about them. So let's go ahead and get started.

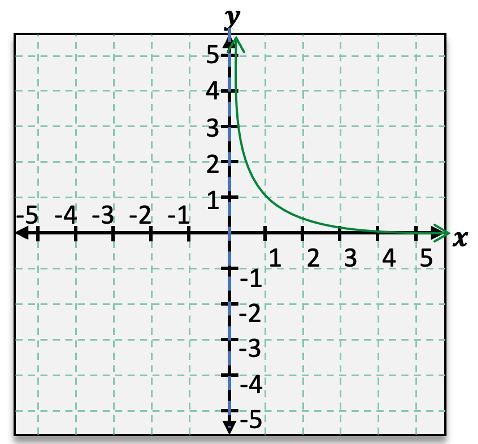
Now the first thing that I want to do here is just go ahead and graph a rational function. This function fx=1x is a really basic and common rational function that you're going to see. So let's go ahead and start with graphing the number 1 and plot those points on our graph. So if I plug in 1 to my function, that just gives me 1 divided by 1, which we know is just 1. Plotting that point on my graph, I have the point (1,1). Then, if I take 2, this is just 1 over 2. So I can also plot that on my graph at (2, 12). Then if I go to 3, this is just 1 over 3. So we're getting even smaller here on our graph. And if I take it all the way to 10 that's going to give me 1/10 or 0.1. We see that these numbers are getting progressively smaller. 1 over 100 is a much smaller number than 1. So what we're going to see happen on our graph is that we're going to get really, really close to the x-axis but not quite touch it. We're going to see the same thing happen on the negatives, but let's go ahead and verify that. I know that if I plug negative 1 in, I'm going to get negative 1. So I can go ahead and plot that point on my graph at (-1, -1). Then if I go to negative 10, I'm going to get negative 1/10, so we know that it's getting smaller in that negative direction. We're going to see a similar thing happen over here.

Now it's getting really, really close to that x-axis but not quite touching it, which is exactly what we said an asymptote is. So what I have here is an asymptote at my x-axis, which I represent using a dotted or dashed line. I'm going to go ahead and highlight it just so that it's easier to see here. But here I have a horizontal asymptote, because it's just a horizontal line, at y equals 0. Whenever we look at this asymptote, we see that our graph is getting really close to it but not quite touching it. And whenever we worked with the end behavior of polynomials, we described the behavior using arrow notation. We said that as x approached infinity, f of x approached something, for this particular graph, it's approaching infinity. And as x approaches negative infinity, here f of x is also approaching infinity.

Now we want to do the same thing with our rational function. Here as x approaches infinity, which is on this side of my graph, I see that f of x is instead approaching my asymptote at 0. So fx→0. Then, on the other side, as x approaches negative infinity, I know that the same thing is happening. I'm still approaching that asymptote at 0. So we can describe our end behavior still with arrow notation, but now it's getting close to that asymptote rather than going to negative or positive infinity.

Let's go ahead and graph the rest of our function, because we still don't know what's happening in the middle here. Now if I take a look at some smaller numbers, between 0 and 1, like, say 0.1, that will give me 1 over 0.1, which is just 10. Then if I get even smaller, even closer to 0, and I take 1 over 0.01, that gets even bigger at 100. We're going to see our graph get really high up and get really close to my y-axis but not quite touch it. The same exact thing is going to happen over here. So I end up having two different curves on the graph of this rational function.

Whenever we consider that it's getting really close to the y-axis, that tells us that we're dealing with another asymptote. So I have an asymptote here on my y-axis, which I will again represent with a dashed line. I'm going to go ahead and highlight it again so that we can see it a little better. And this is a vertical asymptote at the line x equals 0. We see that our graph is approaching this asymptote from either side. And whenever we worked with polynomial functions, if we were to consider x approaching 0 and wanting to describe the behavior of our graph, we would just get a single point, in this case 0. Now, on our rational function, we see that our graph is approaching that line from either side, so that means we need to be able to describe both of these behaviors. We're going to split the behavior into two different sides. As x approaches 0 from the right side, which we denote as x approaching 0 with this little positive, that just means from the right. Here, as x approaches 0 from the right side, I see that my function is getting really, really high. It's approaching infinity. Then, as x approaches 0 from the left side, I see that it's going the opposite direction, so f of x is instead approaching negative infinity.

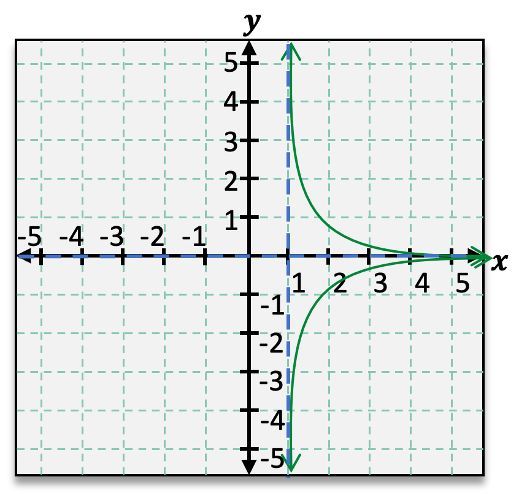
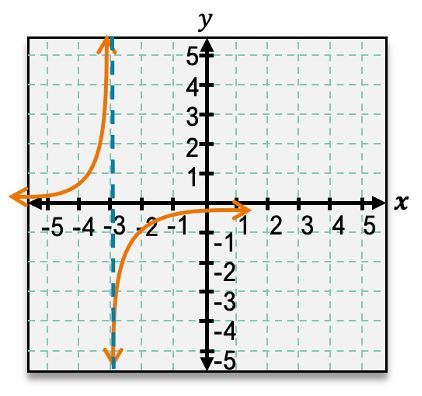
This is only we're only looking at 0 here because that's where our asymptote is and our asymptotes are not always going to be at 0; they just happen to be for this function. So this could really be any number. I could consider x approaching 2 or 5 from either side or any other number. It just depends on your function and where your asymptote is. And there can actually be a lot of different possibilities with asymptotes because a rational function may have no asymptotes. We may have just one asymptote like we see in this function here, or we can even have multiple asymptotes. But they're always going to be represented with these dashed lines. So if we look at function B here, I have a vertical and a horizontal asymptote kind of shifted from what we saw in our function up there. And then I could also even have multiple vertical asymptotes. It really just depends on your function. Now that we know the basics of asymptotes, let's go ahead and get to calculating them. I'll see you in the next video.

Sketch the graph of the function $f\left(x\right)=\frac{1}{x^2}$. Identify the asymptotes on the graph.

• A. Vertical Asymptote: $x=0$, Horizontal Asymptote: None
• B. Vertical Asymptote: $x=0$, Horizontal Asymptote: $y=0$
• C. Vertical Asymptote: $x=0$, Horizontal Asymptote: $y=0$
• D. Vertical Asymptote: $x=1$ , Horizontal Asymptote: $y=0$

Determining Vertical Asymptotes

Hey everyone. Now that we know what asymptotes are, we need to be able to determine where they are on our graph when given a function. So whenever I'm given a rational function, I need to be able to determine if there even is a vertical asymptote and where exactly that vertical asymptote is. Now luckily, finding vertical asymptotes is super simple and almost identical to finding the domain of our function when we set our denominator equal to 0 and solved for x. We're going to do the same thing to find vertical asymptotes, set our denominator equal to 0, and solve for x. But before we do that, we're just going to write our function in lowest terms. So we're going to do two things that we already know how to do in order to find our vertical asymptotes. So let's go ahead and walk through this. So like I said, to find our vertical asymptotes, we want to go ahead and put our function in lowest terms. So looking at the function that I have here, I have x+2 plus 2 times x minus 3. So to put this in lowest terms, I'm going to go ahead and cancel my common factors, which here is x+2. So this leaves me with the function 1x-3, and now my function is in lowest terms. Now I'm simply going to set my denominator equal to 0 and solve for x to get my vertical asymptote. So if I take x minus 3 and I set it equal to 0, I'll end up with x equals 3. And that's my vertical asymptote, and I'm done. That's all we need to do in order to find those vertical asymptotes. Let's go ahead and look at a couple more examples and find some vertical asymptotes of different functions.

So looking at my first function here I have f(x) is equal to 22x+6. So remember the first thing we want to do is write our function in lowest terms. Let's go ahead and do that here and factor. So my numerator cannot be factored; it's just a constant, it's just 2, But in my denominator, I can go ahead and pull out a greatest common factor of 2, and that leaves me with x+3 in my denominator there. So writing this in lowest terms, I'm going to go ahead and cancel these twos, leaving me with 1x+3. Now I can go ahead and set my denominator equal to 0. So setting this denominator equal to 0, isolating x, I'm just going to subtract 3 on both sides, leaving me with x is equal to negative 3. So that's my vertical asymptote of this function and I'm done.

Let's look at one more example here. Here I have f(x) is equal to 1x2-9. Now looking at this function, we want to go ahead and write it in lowest terms but because my numerator is just 1, that's not going to cancel with anything in my denominator. It's already in lowest terms. So I can just go ahead and take my denominator and set it equal to 0. Now solving for x here, I want to add 9 to both sides. Canceling that 9 out, leaving me with x2=9, And then I can go ahead and square root both sides to isolate x, leaving me with x is equal to plus or minus the square root of 9, which we know is just 3. So this tells me that I actually have two vertical asymptotes, one at x equals positive 3 and one at x equals negative 3. It's totally okay to have more than one vertical asymptote. That's going to happen sometimes, and it's perfectly fine. Now that we know how to find vertical asymptotes, let's go ahead and get some more practice.

Based only on the vertical asymptotes, which of the following graphs could be the graph of the given function? $f\left(x\right)=\frac{x^2-4x}{x^2-x-12}$

• A.
• B.
• C.

Determining Removable Discontinuities (Holes)

Hey, everyone. Whenever we find vertical asymptotes, we would factor our function and then cancel out any common factors in order to get our function in lowest terms before setting our denominator equal to 0 and solving for x. But having that common factor actually causes something to happen in the graph of my function called a hole. And we need to be able to identify where these holes are on our graph, which we can do by simply taking our common factor and, instead of canceling it out, just setting it equal to 0 and solving for x. So here, I'm going to walk you through how to find any holes in your graph starting from exactly where we do with vertical asymptotes and just taking a different path from there. So let's go ahead and get started.

Now with vertical asymptotes, like I said, we would cancel out our common factor, and then we would set our denominator equal to 0 and solve for x. So for this function here, I end up with a vertical asymptote at \( x = 3 \), which we can see right here on our graph, a vertical asymptote at \( x = 3 \). Now, whenever we find the holes in our graph, we're going to start at the same place. So here, I have this factored function, but instead of canceling out that common factor to put it in lowest terms, I'm going to take that common factor and set it equal to 0. So here, my common factor is \( x+2 \) and setting that equal to 0, I then want to solve for x. So solving for x here, I end up with \( x = -2 \). And that tells me that I have a hole in the graph of my function right at \( x = -2 \), which I'm going to represent with an open circle on my graph. Literally, just draw a circle on the curve at that point. And that's where our hole is.

So looking at this graph, now I have both a vertical asymptote and a hole for the same function. Now you might also hear holes referred to as removable discontinuities. And that's just the more technical term for it, but it's referring to the same thing. So now that we know how to find the holes in the graph of a rational function, let's go ahead and look at some more examples here.

Let's look at this first function. I have \( f(x) = \frac{x+3}{x^2 + 4x + 3} \). So here, we want to go ahead and factor this the same way we would if we were trying to write it in lowest terms. So my numerator can't be factored here it just stays as \( x+3 \) but my denominator using the ac method can be factored to \( (x+3)(x+1) \). Now I want to take my common factor, and I don't want to cancel it. I want to take it, and I want to set it equal to 0. So here, my common factor is \( x + 3 \). Setting that equal to 0, I'm going to go ahead and solve for x, which I can do by subtracting 3 on both sides. So I end up with a hole at \( x = -3 \) and I'm done. That's where the hole on my graph will be.

Let's look at one more example here. On this side, I have \( f(x) = \frac{x^2 + 1}{x - 1} \). So let's go ahead and factor this. So my numerator can be factored into \( (x+1)(x-1) \) And I'm going to divide that still by \( x - 1 \) because that denominator can't be factored anymore. Now, again, I want to take my common factor, and I don't want to cancel it. I'm going to take and set it equal to 0. So here, \( x - 1 = 0 \). And then we want to solve for x. So adding 1 to both sides leaves me with \( x = 1 \), which is where the hole on my graph is at \( x = 1 \). And we're done. So now that we know how to find the holes in our graph, let's get a little bit more practice.

Find all vertical asymptotes and holes of each function.

$f\left(x\right)=\frac{-5x}{\left(2x-3\right)^2}$

• A. Hole(s): $x=0$ , Vertical Asymptote(s): $x=\frac32$
• B. Hole(s): $x=\frac32$ , Vertical Asymptote(s): $x=\frac32$
• C. Hole(s): $x=0$ , Vertical Asymptote(s): $x=0$
• D. Hole(s): None , Vertical Asymptote(s): $x=\frac32$

Find all vertical asymptotes and holes of each function.

$f\left(x\right)=\frac{x^2-2x}{2x^3-x^2-6x}$

• A. Hole(s): None, Vertical Asymptote(s): $x=0,x=2,x=-\frac32$
• B. Hole(s): $x=0$, Vertical Asymptote(s): $x=-\frac32$
• C. Hole(s): $x=0$, $x=2$, Vertical Asymptote(s): $x=-\frac32$
• D. Hole(s): $x=0$, $x=2$, Vertical Asymptote(s): $x=\frac32$

Find all vertical asymptotes and holes of each function.

$f\left(x\right)=\frac{x^2+10x+25}{2x^2+8x-10}$

• A. Hole(s): None, Vertical Asymptote(s): $x=-5,$ $x=1$
• B. Hole(s): $x=-5$ , Vertical Asymptote(s): $x=1$
• C. Hole(s): $x=1$ , Vertical Asymptote(s): $x=-5$
• D. Hole(s): $x=-5$ , Vertical Asymptote(s): $x=-1$

Determining Horizontal Asymptotes

Hey, everyone. When working with vertical asymptotes, we found that they affect the domain of our rational function, and we could even calculate them in a way that's really similar to finding the domain. Now with horizontal asymptotes, they're instead going to affect the range of our rational function because now we're dealing with horizontal lines that go across our y-axis. Now because we don't have a way of finding the range of our function, you may be worried that this is going to get complicated. But don't worry because it literally just depends on only two things that we can determine by just looking at our function, and that's the degree of both our numerator, the top of our rational function, and the denominator, the bottom of our rational function. So with that in mind, let's go ahead and just jump right into finding some horizontal asymptotes. So looking at this first function here, I have f(x) = \frac{1}{x} and we want to be able to determine what our horizontal asymptote is. Now we're going to look at, like I said, the degree of the numerator and the degree of the denominator. So here the degree of my numerator is 0 because there's not even a variable there, and the degree of my denominator is 1. So 0 I know is less than 1, and whenever the degree of your numerator is less than the degree of your denominator, you are always simply going to have a horizontal asymptote at the line y = 0, which is what we can see on our graph is already happening here. Our line or horizontal asymptote is at y = 0. And that's it. So when your degree of your numerator is less than the degree of your denominator, that's all. Now let's look at one other possibility here. So looking at my function here, I have f(x) = \frac{2x + 3}{x}. So looking at the degree of my numerator and denominator, the degree of my numerator is 1, and the degree of my denominator is also 1. I know that 1 is definitely equal to 1. So here the degree of my numerator is equal to the degree of my denominator. Now whenever that happens, I actually need to look at one more thing on my function, and that is my leading coefficients. So I'm going to take the leading coefficient of my numerator and then divide it by the leading coefficient of my denominator, and that will give me my horizontal asymptote. So here the leading coefficient of my numerator is 2. And the leading coefficient of my denominator is this invisible one here. So my horizontal asymptote is 2 divided by 1, which is just the line y = 2, which we can see is exactly where that horizontal asymptote is on our graph. So now that we know how to find them looking at the degrees of our numerator and denominator, let's go ahead and find some horizontal asymptotes of a couple more functions. So looking at this first example here, I have \frac{4x^{2}}{-x^{3} - 5x + 9}. So looking at the degree of our numerator, I have a 2. And then the degree of my denominator I have is 3. So 2 is less than 3. Well, it's definitely less than. So looking back at our original examples, we know that if our numerator is less than the denominator, y = 0, that will give me my horizontal asymptote. So here, I simply have a horizontal asymptote at y = 0, and I'm done. Let's look at one more example. So here I have f(x) = \frac{2x^{2}}{3x^{2} + x - 1}. So here looking at those degrees, I have a degree of 2 in my numerator and I also have a degree of 2 in my denominator. So the degree of my numerator is definitely equal to the degree of my denominator. Now remember, whenever that happens, we need to take a look at one more thing here, our leading coefficients, in order to find our horizontal asymptote. So the leading coefficient of my numerator is 2 and that of my denominator is 3, taking those and dividing them, I end up with a horizontal asymptote at y = \frac{2}{3}. And that's where my horizontal asymptote will be on my graph. And that's all. So we know how to calculate horizontal asymptotes, but I want to mention one more thing that you might see pop up in your studies. So sometimes, whenever we have a horizontal asymptote, the graph of your rational function may actually intersect it and just fully cross it and then approach it from the other side. Now I know that this goes against what we said our definition of asymptotes are, but it's actually just crossing it and then approaching it from the other side. Now you don't need to worry about why this happens or figuring out when it will. You just need to be aware that it can happen. So don't be afraid when you see a graph, and it crosses a horizontal asymptote. It's totally fine. It can happen. It won't always. But just be aware that it can. So we now know how to calculate horizontal asymptotes and how they may appear on our graph. Let's get some practice.

Find the horizontal asymptote of each function.

$f\left(x\right)=\frac{-5x}{\left(2x+3\right)^2}$

• A. Horizontal Asymptote at $y=0$
• B. Horizontal Asymptote at $y=-\frac54$
• C. Horizontal Asymptote at $y=-\frac52$

Find the horizontal asymptote of each function.

$f\left(x\right)=\frac{8x^2+1}{2x^2-x-6}$

• A. Horizontal Asymptote at $y=0$
• B. Horizontal Asymptote at $y=\frac14$
• C. Horizontal Asymptote at $y=4$

Find the horizontal asymptote of each function.

$f\left(x\right)=\frac{x^2+4x}{2x^3+8x^2}$

• A. Horizontal Asymptote at $y=0$
• B. Horizontal Asymptote at $y=\frac12$
• C. Horizontal Asymptote at $y=2$