Graphs of Tangent and Cotangent Functions - Online Tutor, Practice Problems & Exam Prep

The tangent function graph is derived from the identity \( \tan(x) = \frac{\sin(x)}{\cos(x)} \), featuring asymptotes at odd multiples of \( \frac{\pi}{2} \). Its period is \( \frac{\pi}{b} \), differing from sine and cosine's \( \frac{2\pi}{b} \). The cotangent graph, being the reciprocal of tangent, has asymptotes at integer multiples of \( \pi \) and mirrors the tangent's behavior but inverted. Both graphs repeat every \( \pi \) units, emphasizing their interconnectedness in trigonometric functions.

Introduction to Tangent Graph

Hey, everyone. So in the last few videos, we've been on this journey of looking at the various graphs of trigonometric functions. We've already seen what the sine and cosine look like, as well as what the secant and cosecant look like. Well, in this video, we're now going to take a look at the graph of the tangent function. If this sounds intimidating, don't sweat it because we're going to learn that just like the cosecant and secant function, the tangent function will also build off of the knowledge we've already learned about these graphs like the sine and cosine, for example. So without further ado, let's take a look at what some of these problems might look like in this course where we have to deal with the tangent function as a graph.

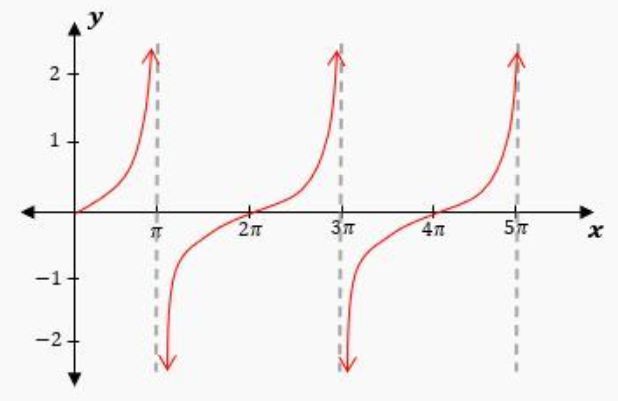
Now I want you to recall this identity which we learned about early on, that says that the tangent of some value is equal to the sine over the cosine. So what we can do is use this to graph what the tangent looks like by taking the sine and dividing it by the cosine values. Now I'm going to go ahead and start with 0, and I know that I'm doing this a bit out of order, I'm not starting from the left side this time, but you're going to see why. So if I start with 0, notice that we're going to have at an x value of 0 that our tangent is sine over cosine. Well, that's just going to be 0 divided by 1, which is 0. So that means we're going to start right about there on our graph.

Now what I'm going to do from here is go to the right and the left on this graph. So start by going to the right at an x value of pi/4. This is going to give you 22 divided by 22, which is just a value of 1. So at pi/4, we have an output of 1 for our tangent graph. Now if I go to the left, we're going to have negative 22 divided by that same value but positive, and doing this will give you a value of negative one, meaning we're going to be down here on our graph.

Now what happens if I go to pi/2 and negative pi/2 on the x-axis? Well, I want you to notice something in both these situations. We end up having this case where we have to divide by 0. We're going to have negative one over 0 and one over 0. We learn in math that you cannot divide by 0, so that means that these values are undefined. And because we get undefined values at negative pi/2 and at pi/2, that means we're going to have asymptotes show up. So we're going to have an asymptote right about here, and an asymptote right about there. And recall that an asymptote just means the function is approaching infinity at that point.

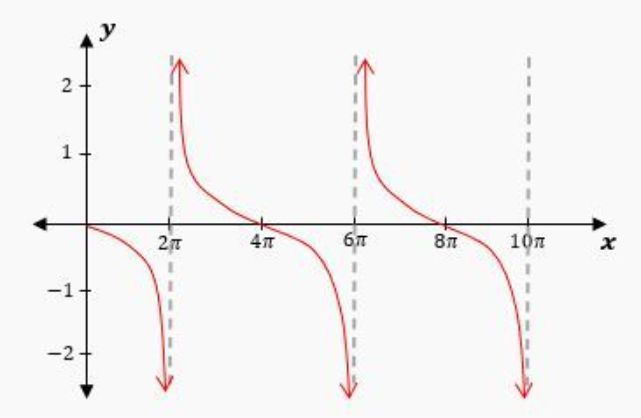
So that means our graph is going to look something like this. We're going to go down and then to the left, and we're going to go up and to the right. So this would be the curve for our tangent function. Now there are a couple of things you should know about the tangent graph. The tangent graph actually has some similarities to the secant graph, which we saw in the previous video. And one of the similarities is that the asymptotes are at the same values on the x-axis. Notice how we have asymptotes at negative 3π/2, negative π/2, π/2, and 3π/2. Well, for our tangent graph, we can see these asymptotes are the same. And it turns out that for the tangent graph, all of the asymptotes or places where the curve repeats are going to be when the cosine of x is equal to 0, which are going to be at odd multiples of π/2. So because of this, on our tangent graph, we're going to see another asymptote at negative 3π/2, and an asymptote at positive 3π/2.

Now the way that the tangent graph is different than the secant graph is, well, obviously this curve is different, but it turns out that for the tangent graph, the tangent graph actually has a period of only pi. Right? For the secant graph, we can see that what happens is we get this smiley face, then this frowny face, and then the curve is going to repeat. So we'll get another smiley, then another frowny. So it repeats after 2 pi units because the distance from here to there on the x-axis would be 2 pi. Well, it turns out that the tangent graph will actually repeat every pi units. So rather than getting some kind of different curve, you're going to get this same curve right about here, and you're going to get this same curve right about there. So every time you travel a distance of pi on the x-axis, you're going to get your curve repeating.

And what this does is actually changes the period for the tangent. So in the past, we've seen that the period for the sine, cosine, cosecant, and secant is 2 pi over b. Well, it turns out when dealing with the tangent, the period is only π/b. So that's something you have to watch out for when dealing with the tangent graph. But the nice thing about the tangent graph is that all of the same transformation rules apply that have been used for sine and cosine aside from the period change.

Now to make sure that we're understanding this, let's try an example where we have to graph the function which deals with the tangent. So here we're asked to graph the function y equals the tangent of π/2 times x. And the way that I'm going to do this is first figuring out what the period of our graph is going to be. Well, recall that the period for tangent, as we just learned, is π/b, rather than 2π/b. And the b value is what we have in front of the x, so we're going to have π divided by π/2. Now what I can do is take this fraction we have in the denominator and flip it and bring it to the numerator. So I can flip this right here, and we're going to get 2π divided by π because this will stay in the denominator, and then those π's are going to cancel giving us a period of 2. So if our period is 2, that means that every 2 units, we're going to have our curve repeat. So what I'm going to do is label the x-axis like this. We're going to say that we have points here 1, 2, 3 on the x-axis, and then we have negative 1, negative 2, negative 3.

Now we've learned that how the tangent graph starts right here at the middle, and then it curves up to the right and down to the left. And we also know that this tangent graph is going to have a period of 2. So what I can do is draw asymptotes here at negative one, and I can draw another asymptote at positive one, because this would be a period of 2 on the x-axis. So what I can do is draw a curve that looks something like this. So this would be the tangent graph. Now because the period is 2, if I start here where this asymptote is, and I go 2 units to the right, we're going to have another asymptote at positive 3. And if I go to negative one, we can go 2 units to the left and get another asymptote at negative 3. And this curve is going to repeat between each of these asymptotes, so we're going to get the same curve right about there and the same curve back here. So this is what the graph would look like, and that is the solution to the problem.

So hopefully this gives you a better understanding of how to work with graphs when you're dealing with the tangent function. Thanks for watching, and please let me know if you have any questions.

Below is a graph of the function $y=\tan\left(bx\right)$. Determine the value of b.

• A. $b=\frac14$
• B. $b=\pi$
• C. $b=2$
• D. $b=\frac12$

Example 1

Hey everyone. So here we have an example where we are asked to graph the function y=12tan⁡x−π2. Now to solve this problem, what I'm going to do is I'm first going to graph the function y=12tan⁡x. So I'm going to ignore this π2 for now, but we will get to this later. So let's start with this function.

To find y=12tan⁡x, well, let's just recall what the tangent graph looks like. I know for the tangent graph, we have asymptotes at negative π2, and at π2. Now we do have more asymptotes on this graph, we're going to have other asymptotes at odd multiples of π2, but for now I'm just going to draw these two.

Now as for the curve, I know the curve starts here in the middle, and then it goes up to the right, and then down and to the left. Now another thing that I recall for this tangent is that we have points at π/4 ,1, and then at negative π/4 ,−1. So the graph ends up looking something like this. But notice that we have a one half in front of our tangent. And this one half, as you may recall, changes the amplitude or tallness of our graph. So rather than having these points at 1 and negative one on the y-axis, these are going to be reduced. So we're then going to have a point instead at one-half, and then at negative one-half for these two values of π/4 and negative π/4 respectively.

So that means the graph is going to look a little bit more like this, where we can see that we're a little shorter than we were before. So this right here would be the curve for y=12tan⁡x.

Now our last step for solving this problem is going to be to incorporate this π2. So we need to figure out what y=12tan⁡x−π2 looks like. Well, something that I can see is that we have a π2 here which is going to shift our graph in some sort of way. And to find the shift we'll recall that we can find the h over b ratio, which tells us how much we've shifted to the left or to the right. Now see that we have a minus sign here, which means that this is actually a positive h value. So we have positive π/2 divided by b, which in this case is any number in front of the x, but since there's nothing there we can just write 1. So we're going to have h over b is equal to just π/2, because this one in the denominator is just going to keep this the same. So that means that our graph is going to be shifted π/2 units to the right. So what I can do is take this point right here, and I can shift it π/2 units to the right.

Introduction to Cotangent Graph

Welcome back everyone. So at this point, we should be familiar with the graphs for most of the trigonometric functions. We've talked about the sine and cosine graphs, the secant and cosecant, we even looked at the tangent graph in the previous video. Now last but not least, we're going to be taking a look at the cotangent graph. And this might sound a bit complicated just because learning about another trigonometric graph might feel kind of exhausting at this point, but what we're going to learn is that the cotangent graph is actually closely related to the tangent graph. And if you recognize how these two are related, then sketching this graph is actually pretty straightforward. So without further ado, let's get right into things.

Now recall how based on reciprocal identities, the cotangent is just one over the tangent. So we can use this fact to draw the graph for the cotangent. Now the first thing that I'm going to look for is where we can draw these asymptotes because this will tell us how wide each of the cycles of our graph is. Now if I look at the tangent graph, I can see that we reach 0 at negative π, 0, and π. So we reach 0 on the y-axis at all these points. And it is a fundamental math rule that you cannot divide by 0. So any place that we see 0 on the tangent is going to be a value that cannot exist on our cotangent graph, so that's going to be where we draw the asymptotes. So there's going to be an asymptote right here at negative π. There's also going to be an asymptote here at 0 on the x-axis, and there's going to be an asymptote over here at positive π.

Now from here, we need to draw the curves. And it turns out that the curves for the cotangent graph are similar to the tangent graph, but they're going to be flipped upside down. So rather than having curves that go from bottom left to top right, we're going to have curves that go from top left to bottom right. So the graph is going to look something like this.

Now I want you to notice all the points on the cotangent graph where we touch the x-axis. This is where our output is going to be equal to 0. And we can actually see that these values make sense when we look at the tangent graph because notice that we touch the x-axis at negative 3π/2. And on our tangent graph, our graph gets really really small when we get to negative 3π/2 in the negative direction. So basically we go to negative infinity. And whenever you divide a number that is approaching infinity, whether it's a negative or positive number, then your entire fraction or output is going to be equal to 0 approximately, or it's going to approach 0. So that is why we have 0 at negative 3π/2, at negative π/2, at π/2, and at 3π/2. So you can see at all of these points on our tangent graph, we're either getting really, really big there towards infinity or really small towards negative infinity.

Now one of the main similarities between the tangent and cotangent graph is the distance on our x-axis, which we have the curves repeat. Because notice for our tangent graph that we repeat from negative π/2 to π/2, which is just a distance of π, and it is a distance of π for each of these curves on this graph. So that is one of the nice things about the tangent graph—it repeats every π units, and it turns out that the cotangent graph is the exact same thing. Because if you look, we can see here our graph goes from negative π to 0, and that's just the distance of π. And we also go from 0 to π, which is also a distance of π. So it turns out that this graph repeats every π amount of units as well.

Now because these graphs have the same repeating behavior, it turns out their periods are also going to be the same. So just like the period of the tangent was π/b, the period of the cotangent is also π/b.

Now the way these graphs are different from each other is the values of their asymptotes. For example, if we take a look at the tangent graph, we can see that these asymptotes will always show up at odd multiples of π/2. So notice we have an asymptote at negative 3π/2, negative π/2, we have an asymptote of π/2, 3π/2. Then if we kept extending this graph, we would also have asymptotes at 5π/2 and 7π/2. Now notice for our cotangent graph, our asymptotes are at negative π, they are at 0, They are at π. If we kept extending this graph, we would see asymptotes at 2π, 3π, 4π. So it turns out for the cotangent graph, the asymptotes are always going to be at integer multiples of π. So the location of the asymptotes on the x-axis, as well as the fact that these curves are flipped upside down, are really the two main differences between the cotangent and tangent graph.

Now to make sure we understand this concept well, let's try an example where we have to deal with the cotangent. Now to solve this problem, what I'm first going to do is figure out where the asymptotes are going to be located on this graph, and I can do that by looking at what our cotangent is. Now recall that the period of the cotangent is just π/b. Now what I can see here is that our b value is the value we have in front of the x, And I can see that b value is equal to π, so we're going to have π/π. And whenever you divide one number by itself, it's just going to be equal to 1, because they're both going to cancel and give you one. So that means that our period is equal to 1. So what I can do on our graph is I can draw points every one unit on our x-axis. So I'll go 1, 2, 3 to the right, and that'll go negative 1, negative 2, negative 3 to the left. Now the period of this graph is 1, meaning I can draw these asymptotes for every one unit. So we're going to have an asymptote here at 0, we're going to have it at 1, and then at 2, and then at 3. And then these asymptotes are also going to repeat behind the graph as well. So I have an asymptote at negative one, negative two, negative three, and this is what the asymptotes are going to look like.

Now my last step for solving this problem is going to be to draw the curves. And recall that the curves go from top left to bottom right between these asymptotes. So what I'm going to do is have a curve that looks something like that, and these curves are just going to keep repeating for each period of this graph because that's what the repeating function is going to do. So this right here is the graph of our function and the solution to this problem.

So that is how you can deal with graphs when it comes to the cotangent function. Hope you found this video helpful. Thanks for watching, and please let me know if you have any questions.

Below is a graph of the function $y=\cot\left(bx+\frac{\pi}{2}\right)$. Determine the value of b.

• A. $b=\frac14$
• B. $b=1$
• C. $b=2$
• D. $b=\frac12$

Example 1

Hey, everyone. So let's see how we can go about solving this example. So here we are asked to graph the function y=-2cot⁡(x4). Now, what I noticed in this problem is we have quite a few things going on. We have a negative sign here, which is going to cause our graph to be flipped or reflected over the x-axis. I also notice we have this 2 here, which is going to change the amplitude or tallness of our graph. And we have this 14 on the inside of our cotangent, which is going to change the period. So where do we even get started with this? Well, what I like to do is start with what I'm familiar with. And what I'm going to do is I'm going to ignore this amplitude and negative sign out front. I'm just going to focus on y=cot⁡(x4). And the reason that I'm focusing on this is because I know how to deal with the period of a cotangent, and I know what the general cotangent graph looks like, so I can build off of something.

Now the period for a cotangent graph is going to be πb, where b is the number that is in front of the x. Since I can see that that's 1/4, I get that we have π/14. You can flip this fraction and bring it to the top, which is going to give you 4π over 1, which is just 4π. So the period is 4π. And something that I know about the cotangent graph is the cotangent graph has an asymptote that starts at an x value of 0. So since we started an x value of 0 and our period is 4π when we have this b value in front of x, that means that starting at 0, we're going to repeat every 4π units. So I can draw another asymptote here at 4π, and I can draw another asymptote over there at 8π.

Now something I know about the cotangent graph is that it goes up into the left, and then goes down into the right, and it reaches 0 on the x-axis right in between the two asymptotes in the middle. Now what you should typically see with a cotangent graph is you're going to see points that are in between these two values, and in between those two values. So this over here should be π, and this should be 3π, and that makes sense do we have π, 2π, 3π, 4π, and at these values you should see an output of 1. So what we should have is an output right about there, which would be at 1, and we should see an output right here, which would typically be at -1. So adjusting our curve a little bit, this is what the function would look like for the cotangent of x4, and then you would get this curve continuously repeating as we go along. But this is not what we're trying to find. We're not trying to find the cotangent of x4.

We're trying to find -2 times the cotangent of x4. Now this number out in front here, the 2, this is going to change the amplitude or basically vertically stretch our graph. So rather than having an output of 1 when you're at π, you should actually have an output of 2 at this value. And then rather than having an output at -1 for 3π, you should actually have an output at -2. So adjusting our curve, the graph should look a bit more stretched vertically when we incorporate this 2. But there's one more thing we need to change about our graph, and that's the negative sign. This negative sign is going to take our whole graph, and it's going to flip it over the x-axis. So doing this, we actually need to, in essence, reverse these two points on the y-axis. So we should have a point right here at π-2, and a point right here at 3π positive 2. So for the graph y=-2cot⁡(x4), it should look something like this where we start at the lower left, and then we go ahead and go up reach a 0 on the y-axis at 2π, and then we come up here and go to the top right. So this is what our curve should look like, and because the cotangent is a repeating graph as we've learned in previous videos, this curve should continue happening between each of these asymptotes. So this would be the graph of our function and the solution to the problem. So hope you found this video helpful, thanks for watching.

Here’s what students ask on this topic:

What are the key characteristics of the tangent function graph?

The tangent function graph, derived from the identity $\tan \left(x\right)=\frac{\sin }{\left(}$, features several key characteristics. It has vertical asymptotes at odd multiples of $\frac{\pi }{/}$ (e.g., $\frac{\pi }{/}$, $\frac{3\pi }{/}$). The period of the tangent function is $\frac{\pi }{/}$, where $b$ is the coefficient of $x$. The graph repeats every $\pi$ units and passes through the origin, with the curve moving from the bottom left to the top right between asymptotes.

How do you graph the cotangent function?

To graph the cotangent function, recall that $\cot \left(x\right)=\frac{1}{/}$. Start by identifying the asymptotes, which occur at integer multiples of $\pi$ (e.g., $0$, $\pi$, $\mathrm{2\pi }$). The period of the cotangent function is $\frac{\pi }{/}$, where $b$ is the coefficient of $x$. The graph repeats every $\pi$ units. The curves of the cotangent graph move from the top left to the bottom right between asymptotes, opposite to the tangent graph.

What is the period of the tangent function?

The period of the tangent function is given by $\frac{\pi }{/}$, where $b$ is the coefficient of $x$ in the function $\tan \left(\mathrm{bx}\right)$. This differs from the sine and cosine functions, which have a period of $\frac{\mathrm{2\pi }}{/}$. For example, if the function is $\tan (\frac{\pi }{/}$, the period would be $\frac{\pi }{/}$, which simplifies to 2.

How do the asymptotes of the tangent and cotangent functions differ?

The asymptotes of the tangent and cotangent functions differ in their locations on the x-axis. For the tangent function, asymptotes occur at odd multiples of $\frac{\pi }{/}$ (e.g., $\frac{\pi }{/}$, $\frac{3\pi }{/}$). For the cotangent function, asymptotes occur at integer multiples of $\pi$ (e.g., $0$, $\pi$, $\mathrm{2\pi }$). This difference is due to the reciprocal relationship between the tangent and cotangent functions.

What is the relationship between the tangent and cotangent graphs?

The tangent and cotangent graphs are closely related but have distinct characteristics. The tangent function, $\tan \left(x\right)$, has vertical asymptotes at odd multiples of $\frac{\pi }{/}$ and repeats every $\pi$ units. The cotangent function, $\cot \left(x\right)$, being the reciprocal of tangent, has vertical asymptotes at integer multiples of $\pi$ and also repeats every $\pi$ units. The cotangent graph is essentially the tangent graph flipped upside down, with curves moving from the top left to the bottom right between asymptotes.